Explanations 
Flashcards 
StudySmarter AI 
Notes 
Study Plans 
Study Sets 
Exams 
Magazine 
Find a job 
Mobile App 
Jump to a key chapter
In this article, we will be learning about the De Broglie hypothesis and see how matter can behave as more than just a particle.
- This article covers the De Broglie hypothesis
- First, we will learn what the De Broglie hypothesis is and what matter waves are
- Next, we will learn how to use the De Broglie wavelength equation
- Lastly, we will learn how the De Broglie hypothesis applies to electrons
De Broglie Hypothesis
De Broglie hypothesized that matter acts as a wave
De Broglie came up with the idea that matter acts like a wave in 1924. Another name for this theory is De Broglie's matter waves.
Matter Waves
Matter waves are the wave-like behavior exhibited by matter
Matter waves are an important part of the theory of quantum mechanics because they show how waves and particles can exist at the same time. Every kind of matter moves in waves. A beam of electrons, for example, can be bent in the same way that a beam of light or a water wave can. Most of the time, though, the wavelength is too small to have a real effect on daily life.
Below is what these matter waves look like:
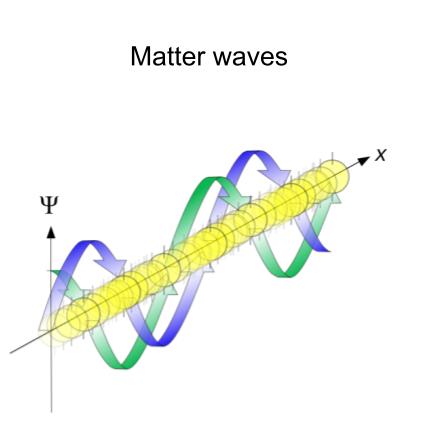Fig.1: An example of a matter wave
The green and blue arrows show the wave-like behavior of a species, while the yellow ball represents the particle-like behavior.
When trying to find the location of a particle at a given point x, the probability of the particle being at "x" is spread out like a wave, as shown above; There is no definite position of the particle.
The probability distribution is shown by the opacity, At the points where the arrow is clearer, there is a lower probability of the particle being there
De Broglie Wavelength equation
As part of his hypothesis, De Broglie proposed an equation for finding the wavelength of a particle.
The De Broglie wavelength equation is used to calculate the wavelength of a particle that exhibits wave-like behavior. The equation is:
$$\lambda=\frac{h}{p}$$
Where:
- λ is the wavelength.
- h is Planck's constant (6.626 x 10-34 \(\frac{kg*m^2}{s}\)).
- p is the momentum.
The de Broglie wavelength, is the wavelength of a particle with mass, as opposed to a particle without mass.
Recall that the formula for momentum (p) is \(p=mv\). Where m is mass and v is velocity. Based on this, the larger the particle, the smaller the wavelength. Because of this, the wavelength of everyday objects is very tiny, so their wave properties are negligible. However, for small particles like protons and electrons, the wavelength is large/important.
Thermal De Broglie Wavelength
The thermal de Broglie wavelength (λ th) is about the average de Broglie wavelength of the gas particles in an ideal gas at the given temperature.
An ideal gas is an estimation of the behavior of a "real" gas. Ideal gases have a negligible volume and mass.
The expression gives the thermal de Broglie wavelength:
$$\lambda_{th}=\frac{h}{\sqrt{2*\pi*m*k_{B}*T}}$$
Where:
- h is Planck's constant.
- m is the mass of the gas particle.
- kB is the Boltzmann constant (1.38 x 10-23 J/K).
- T is the temperature of a gas.
Calculate De Broglie Wavelength
Now that we know what the wavelength equation is, let's put it to use, shall we?
Calculate the De Broglie wavelength of a proton moving at 1.20 x 106 m/s. The rest mass of a proton is 1.67 x 10-27 kg.
Firstly, we need to calculate the momentum:
$$p=m*v$$
$$p=(1.67x10^{-27}\,kg)(1.20x10^6\frac{m}{s})$$
$$p=2.004x10^{-21}\frac{kg*m}{s}$$
Now we can plug this into the wavelength equation:
$$lambda=\frac{h}{p}$$
$$\lambda=\frac{6.626x10^{-34}\frac{kg*m^2}{s}}{2.004x10^{-21}\frac{kg*m}{s}}$$
$$\lambda=3.31x10^{-7}\,m$$
$$1x10{-9}\,m=1\,nm$$
$$3.31x10^{-7}\,m*\frac{1\,nm}{1x10^{-9}\,m}=331\,nm$$
For reference, purple light is about 380 nm, so this wavelength is a bit too small to be visible by the human eye.
De Broglie's Wavelength for Electrons
When electrons orbit the nucleus, the De Broglie waves form a closed loop. The electrons can only exist as standing waves that "fit" in the electron cloud and are "allowed", as shown below:
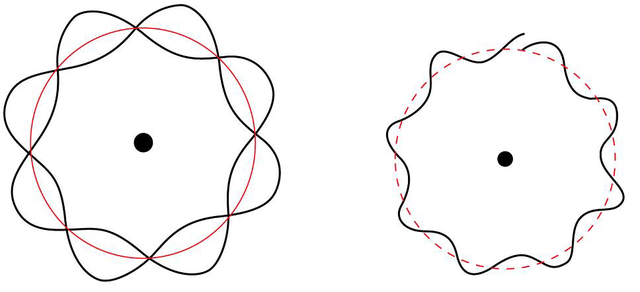Fig.2: An allowed energy level (left) and one that is not allowed (right)
On the left, the standing wave fits along the electron cloud, so it is at an allowed energy level. On the right, the wave doesn't fit and is therefore not at an allowed energy level.
Basically, electrons are allowed to exist at set, quantized energy levels called "shells":
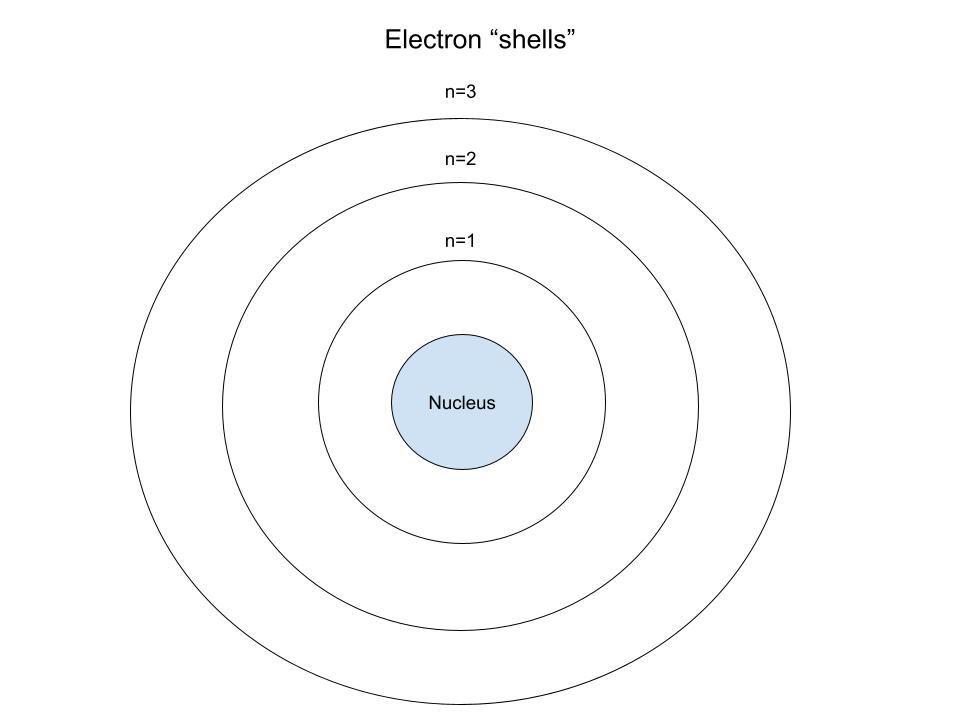Fig.3: Allowed energy levels, also called shells
The closer an electron is to the nucleus, the lower in energy it is. The electrons at the n=1 state are called ground-state electrons, since they are at the lowest energy level.
Now that we've covered how electron waves behave, let's calculate the wavelength!
A ground state electron (n=1) is orbiting a hydrogen nucleus at 2.18 x 106 m/s. The mass of an electron is 9.11 x 10-31 kg. What is the wavelength of this electron?
Like before, we need to first calculate the momentum:
$$p=m*v$$
$$p=(9.11x10^{-31}\,kg)(2.18x10^{6}\frac{m}{s})$$
$$p=1.99x10^{-24}\frac{kg*m}{s}$$
$$\lambda=\frac{h}{p}$$
$$\lambda=\frac{6.626x10^{-34}\frac{kg*m^2}{s}}{1.99x10^{-24}\frac{kg*m}{s}}$$
$$\lambda=3.33x10^{-10}\,m$$
$$\lambda=0.333\,nm$$
Applications of the De Broglie Wavelength
1. The wave properties of matter can only be seen in very small things. Using electrons as the source, a de Broglie wavelength interference pattern can be made. The average energy of an electron in an electron microscope is 10 eV, so the de Broglie wavelength is 3.9 x 10-10 m.
This is similar to how far apart atoms are. So, a crystal acts as an electron diffraction grating. The crystal structure can be figured out by looking at the diffraction pattern.
2. The wavelength used in a microscope limits the size of the smallest things we can see. The shortest wavelength of visible light is 400 nm, which is equal to 4 x 10-7 m. Most electron microscopes use wavelengths that are 1,000 times smaller and can study very small details.
De Broglie Wavelength - Key takeaways
- De Broglie hypothesized that matter acts as a wave
- Matter waves are the wave-like behavior exhibited by matter
- The De Broglie wavelength equation is used to calculate the wavelength of a particle that exhibits wave-like behavior. The equation is:
$$\lambda=\frac{h}{p}$$
- λ is the wavelength
- h is Planck's constant (6.626 x 10-34 \(\frac{kg*m^2}{s}\))
- p is the momentum
- When electrons orbit the nucleus, the De Broglie waves form a closed loop. The electrons can only exist as standing waves that "fit" in the electron cloud and are "allowed"
References
- Fig.2- An allowed energy level (left) and one that is not allowed (right) (https://upload.wikimedia.org/wikipedia/commons/thumb/0/00/Standing_wave_electron_cloud.png/640px-Standing_wave_electron_cloud.png) by CK-12 Foundation (https://ck12.org/) licensed by CC BY-SA 3.0 (https://creativecommons.org/licenses/by-sa/3.0/)
Learn faster with the 10 flashcards about De Broglie Wavelength
Sign up for free to gain access to all our flashcards.
Frequently Asked Questions about De Broglie Wavelength
How to calculate de Broglie wavelength?
The formula for the de Broglie wavelength is:
$$\lambda=\frac{h}{p}$$
Where:
- \(\lambda\) is wavelength
- h is Planck's constant
- p is momentum
When to use de Broglie wavelength?
The de Broglie wavelength best applies to subatomic particles like protons or electrons. The wavelength's of everyday objects are incredibly small, so the wavelength's effect/behavior is negligable.
What does de Broglie wavelength depend on?
The de Broglie wavelength depends on:
- Mass of particle
- Velocity of particle
What is de Broglie wavelength associated with?
De Broglie's wavelength is associated with matter waves, which is the wave-like behavior of matter.
Does de Broglie wavelength depend on temperature?
De Broglie's wavelength indirectly depends on temperature. The de Broglie wavelength depends on velocity, which can fluctuate based on temperature.
About StudySmarter
StudySmarter is a globally recognized educational technology company, offering a holistic learning platform designed for students of all ages and educational levels. Our platform provides learning support for a wide range of subjects, including STEM, Social Sciences, and Languages and also helps students to successfully master various tests and exams worldwide, such as GCSE, A Level, SAT, ACT, Abitur, and more. We offer an extensive library of learning materials, including interactive flashcards, comprehensive textbook solutions, and detailed explanations. The cutting-edge technology and tools we provide help students create their own learning materials. StudySmarter’s content is not only expert-verified but also regularly updated to ensure accuracy and relevance.
Learn more