Energy Diagrams

Total Energy Diagram

"What is energy?" There are many different answers to this question as energy is a concept that can have different meanings depending upon the situation:

• Energy is a way of keeping track, mathematically, of potential and kinetic energy in a physical system.

• A physical system is composed of physical objects that, individually or as a whole, have the capacity to change state.

• The state of a system is determined by the static configurations of objects (potential energy) at any given moment and the movements of objects (kinetic energy) at any other moment.

• The balance between potential and kinetic energy, for a system, is called the total energy, or just energy.

Energy can be described using an operator called the Hamiltonian:

$$\hat{H}=\hat{T}+\hat{V}$$

Where:

• \(\hat{H} \) is the total energy
• \(\hat{T} \) is kinetic energy
• \(\hat{V} \) is potential energy.

You can think of energy as a fluid divided between two containers. Imagine that as the total volume of the fluid is transferred between two containers, one representing potential energy and the other representing kinetic energy. This "energy fluid", is never diminished during the transfer. The total energy of the system is symbolized by \(\hat{H} \).

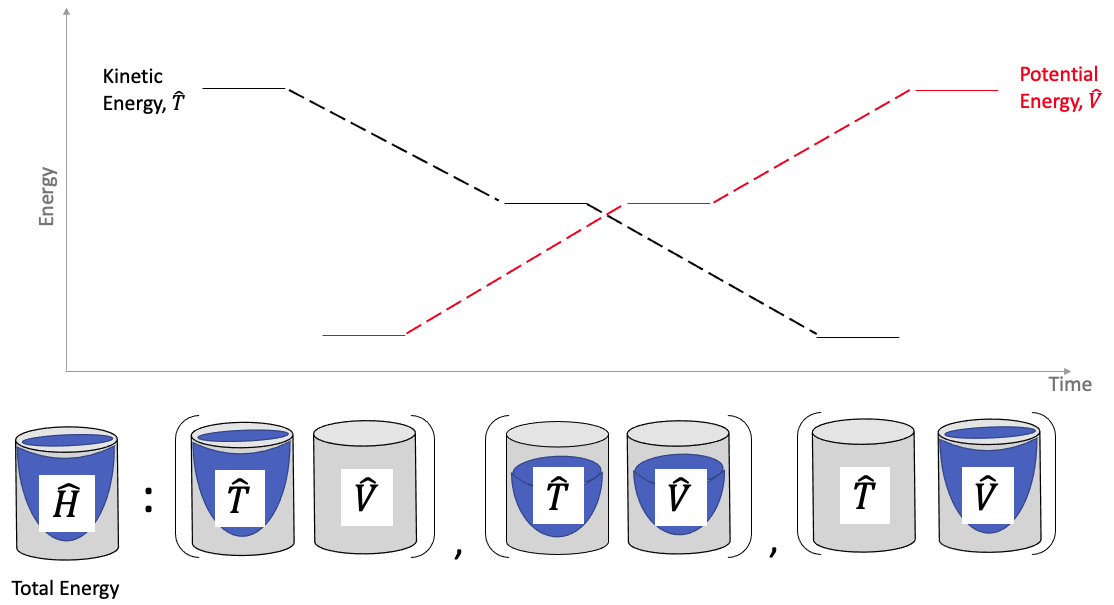Figure 1: Hamiltonian as an energy fluid being transferred between two containers; one holding kinetic energy and the other holding potential energy.

Energy Level Diagram

Now that we have a basic understanding of energy, let's move on to talking about energy levels diagrams. But first, we need to cover some basic definitions.

Now we can ask,

1. "Which energy transformation is represented in the energy diagram?"

• In an energy level diagram, the transformation of total energy, \(\hat{H} \), between potential energy, \(\hat{V} \), and kinetic energy, \(\hat{T} \), over time is represented.

2. "What is the basis for analyzing an energy transfer diagram?"

• To analyze an energy transfer diagram we look at the energy levels (y-axis) and map these to changes in the system over time (x-axis).

• Representations of energy levels on the diagram can be given in terms of orbital energies, enthalpy and potential energy.

• Representations of time on the diagram can be given in terms of the reaction pathway, the reaction coordinate or the coordinates of atoms (potential energy that is frozen in time) within a molecule.

3. "How to draw an energy diagram?"

• To draw an energy level diagram we put a representation of energy levels on the vertical axis and a representation of time on the horizontal axis.

Orbital Energy Diagram

As I just mentioned, energy in an energy level diagram can be represented in different ways. Firstly, let's look at diagrams that represent orbital energies.

1. First let's ask, "What is an orbital energy diagram?" An orbital energy diagram visualizes electron orbitals, in atoms and molecules, in terms of energy levels and electron occupancy. To create an orbital energy diagram we:

• According to the Aufbau Principle, first fill the lowest energy orbitals with electron pairs before we move on to higher energy orbitals.

• According to the Pauli Exclusion Principle, a maximum of two electrons can occupy any one orbital.

• According to Hund's Rule, when there are two or more orbitals that are at the same energy level, each orbital gets one electron first and then any leftover electrons are added to singly occupied orbitals.

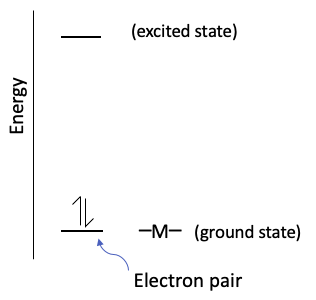Figure 2: Orbital energy diagram, where, -M-, represents a molecule and the energy levels, "ground state" and "excited state", represent orbitals within a molecule.

Example: Let's create an orbital energy diagram for the aluminum atom.

An atom of aluminum has the following electron configuration: 1s²2s²3p⁶3s²3p¹, (13 electrons). Then the orbital energy diagram for aluminum would be:

Figure 3: Orbital energy diagram of Aluminum.

Notice, first that the orbital energy levels, from lowest to highest, are: 1s < 2s < 2p < 3s < 3p. Then, we filled all of the lower energy orbitals (\(1s \rightarrow 3s\)), with pairs of electrons. That left us with one electron that we placed in any one of the 3p orbitals.

Potential Energy Diagram

Now let's ask, "Which diagram represents the potential energy of an exothermic or endothermic reaction?" The answer is that both are represented by a potential energy diagram in terms of the enthalpy of formation for a given chemical reaction.

In terms of the enthalpy of formation for a chemical reaction:

• A potential energy diagram is a graph with enthalpy (potential energy) on the vertical axis and the reaction pathway (time) on the horizontal axis.

Consider the following enthalpy, or potential energy, diagrams for a set of hypothetical chemical reactions:

i. An exothermic chemical reaction:

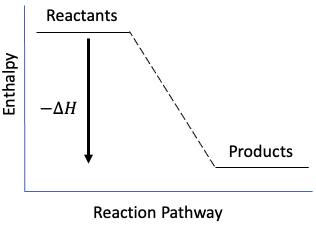Figure 4: Enthalpy diagram for an exothermic chemical reaction. Heat is released by the system, -ΔH.

ii. An endothermic chemical reaction:

Figure 5: Enthalpy diagram for an endothermic reaction. Heat is absorbed by the system, +ΔH.

The above energy diagrams graph the enthalpy, or potential energies, associated with products and reactants. Reactants change to products through a reaction pathway that involves the addition of kinetic energy, in the form of heat, to the system. As noted previously, the enthalpy of the formation of a chemical substance can be viewed as being equivalent to the potential energy that is stored as heat within the chemical bonds of a compound.

Endothermic Energy Diagram

What is an endothermic energy diagram?

Example 1: Let's consider the enthalpy of formation of hydrogen iodide, HI (g), gas from hydrogen gas, H₂ (g), and crystalline iodine, I₂ (s), under standard conditions :

$$H_2 \left( g \right)+I_2 \left( s \right) \rightarrow 2HI \left( g \right)$$

By referring to an appropriate table of thermodynamic data, we can find the enthalpy of formation of the reactants under standard conditions:

• H (g): \(\Delta{H_f}^\circ=+218\,kJ/mol \)

• I (g): \(\Delta{H_f}^\circ=-197.7\,kJ/mol \)

Notice that the enthalpy of formation of elemental hydrogen gas, H₂ (g), is equal to zero - H₂ (g) : \(\Delta{H_f}^\circ=0\,kJ/mol \). However, this reaction initiates with the breaking of molecular hydrogen bonds, which results in the formation of atomic hydrogen gas, H (g) : \(\Delta{H_f}^\circ=+218\,kJ/mol \), and the same is also true for molecular Iodine gas, I₂ (g).

The enthalpy of formation of the elemental form of iodine (crystalline) is, I₂ (s): ΔH_f ° = 0.0 kJ/mol. Again, this reaction involves the sublimation and the breaking of the bonds within molecular the gas, forming iodide gas; I ^- (g): ΔH_f ° = -197.7 kJ/mol.

As such, the actual reaction process is given by:

$$H_2 \rightarrow 2H$$

$$I_2 \rightarrow 2I$$

Substituting this we get:

$$2H + 2I \rightarrow 2HI$$

Notice, we must account for the stoichiometric coefficients of the balanced equation, to get the enthalpy of formation of reactants, such that:

2 ΔH_f ° (Atomic Hydrogen gas, H ) + 2 ΔH_f° (Iodide gas, I ^- ) = 2 · (218.0 kJ/mol) + 2 · (-197.7 kJ/mol) = 40.6 kJ/mol

Thus, the enthalpy of formation for the production of 2 moles of hydrogen iodide, HI, is given by:

2 ΔH_f ° (Hydrogen Iodide, HI )= 40.6 kJ/mol

The enthalpy diagram for this reaction is:

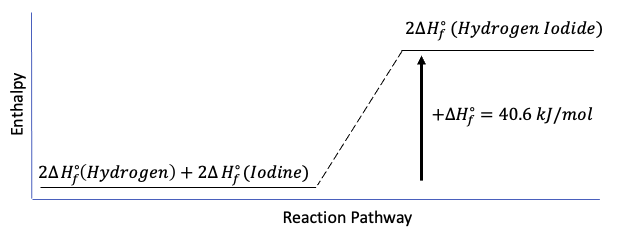Figure 6: Enthalpy diagram, hydrogen iodide synthesis, endothermic reaction.

The synthesis of Hydrogen Iodide from Hydrogen and Iodine is an endothermic reaction that absorbs energy as heat from the surroundings.

Exothermic Energy Diagram

What is an exothermic energy diagram?

• An exothermic energy diagram is a graph with enthalpy (energy) on the vertical axis and the reaction pathway on the horizontal axis.

• An exothermic energy diagram depicts the energy difference between reactants and products. It shows in the release of energy into the surroundings associated with the formation of products.

Example 2: Now let's calculate the enthalpy of formation of nitric oxide, NO , gas from ammonia gas, NH₃, and oxygen gas, O₂ , under standard conditions:

$$4NH_3 \left( g \right)+5O_2 \left( g \right) \rightarrow 4NO \left( g \right)+H_2O \left( g \right)$$

By referring to a standard table for the enthalpies of formation, we are given the enthalpy of formation of the reactants:

• NH₃ (g): ΔH_f° = -45 kJ/mol

• O₂ (g) : ΔH_f° = 0.0 kJ/mol

Notice, we must account for the stoichiometric coefficients of the balanced equation, to get the enthalpy of formation of reactants, such that:

$$Reactants:\,4\Delta{H_f}^\circ{(Ammonia\,gas)}+5 \Delta{H_f}^\circ{(Oxygen\,gas)}=4\cdot (-45.9\,kJ/mol)+5\cdot (0.0\,kJ/mol)$$

$$=-229.5\,kJ/mol$$

However, we must also calculate the enthalpy of formation of products to get the enthalpy of the overall process, ΔH°. Referring to a standard table for the enthalpies of formation:

• NO (g) : ΔH_f° = 90.3 kJ/mol,

• H₂O (g) : ΔH_f° = -241.8 kJ/mol.

Then:

$$Products:\,4\,\Delta{H_f}^\circ\,{(Nitric\,Oxide,\,gas)}+6\,\Delta{H_f}^\circ\,{(H_2O,\,vapor)}=4\cdot (90.3\,kJ/mol)+6\cdot (-241.8\,kJ/mol)$$

$$=-1089.6\,kJ/mol$$

Thus, the overall enthalpy of the process, which is also referred to as the enthalpy of reaction, ΔH °, yielding the production of 4 moles of nitric oxide, NO, is given by:

$$\Delta{H}^\circ =\Sigma\,n\,\Delta{H_f}^\circ (Products)-\Sigma\,m\,\Delta{H_f}^\circ (Reactants)=-1089\,kJ/mol-(-229.5\,kJ/mol)=-906.0\,kJ/mol$$

Where,

• Σ, is the summation symbol

• n and m, are the stoichiometric coefficients of the balanced equation for products and reactants, respectively.

The enthalpy diagram for this reaction is:

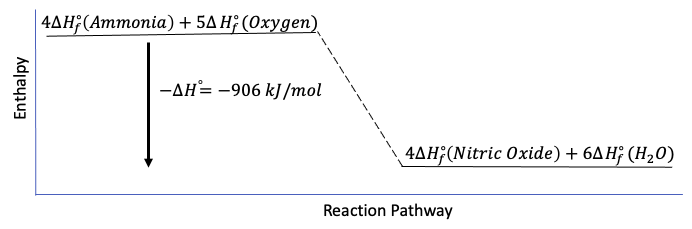Figure 7: Enthalpy diagram, nitric oxide synthesis, exothermic reaction

The synthesis of nitric oxide from ammonia and oxygen gas is an exothermic process.

Energy Flow Diagram

Energy flow diagrams depict how energy (chemical, physical, heat, electric, light, etc.) is transferred and/or transformed in processes within systems. In terms of thermodynamic processes, energy flow as heat is the focus.

For example, imagine that an engine is supplied energy from a heat source, part of which is transformed into useful work. The rest of the energy, that is not used to do work, is lost to the surroundings.

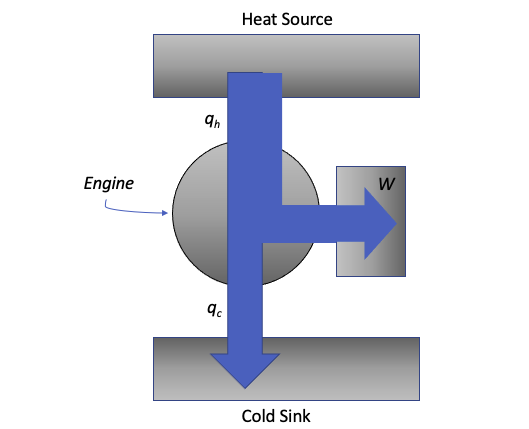Figure 8: Energy Flow Diagram - An engine is supplied energy, q_h , from a heat source, part of which is transformed into useful work, W, with the rest of the energy, q_c , being lost into the cold sink.

This example of an engine is commonly used in thermodynamics and the energy flow depicted above is the basis of many thermodynamic processes.