You might have seen how to approximate the area below curves using different methods. The most common way of approximating areas is by using rectangles. This is what we know as a Riemann sum.
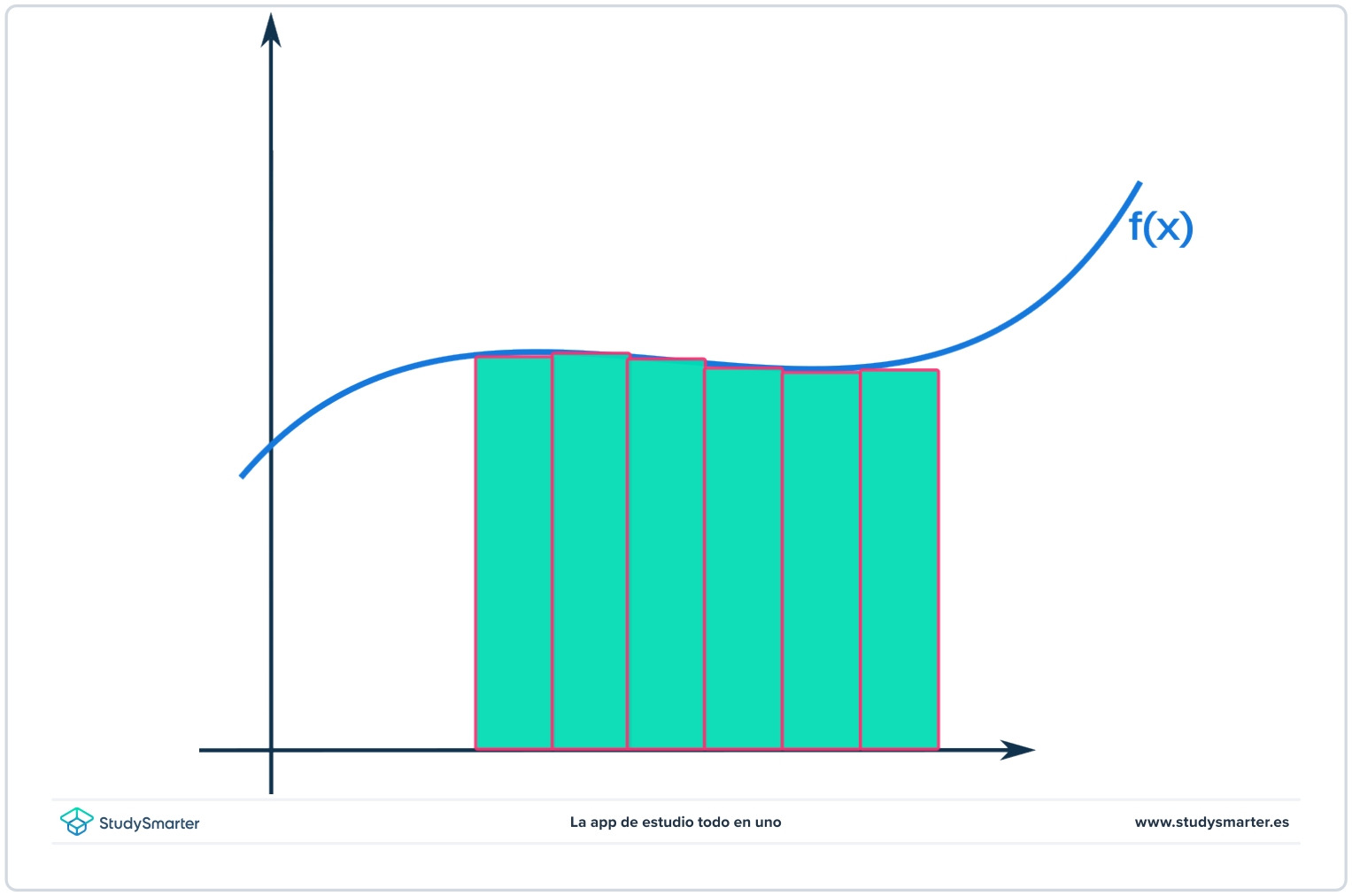
Fig. 1. Approximation of the area below a curve using rectangles.
But what about the exact area below a curve?
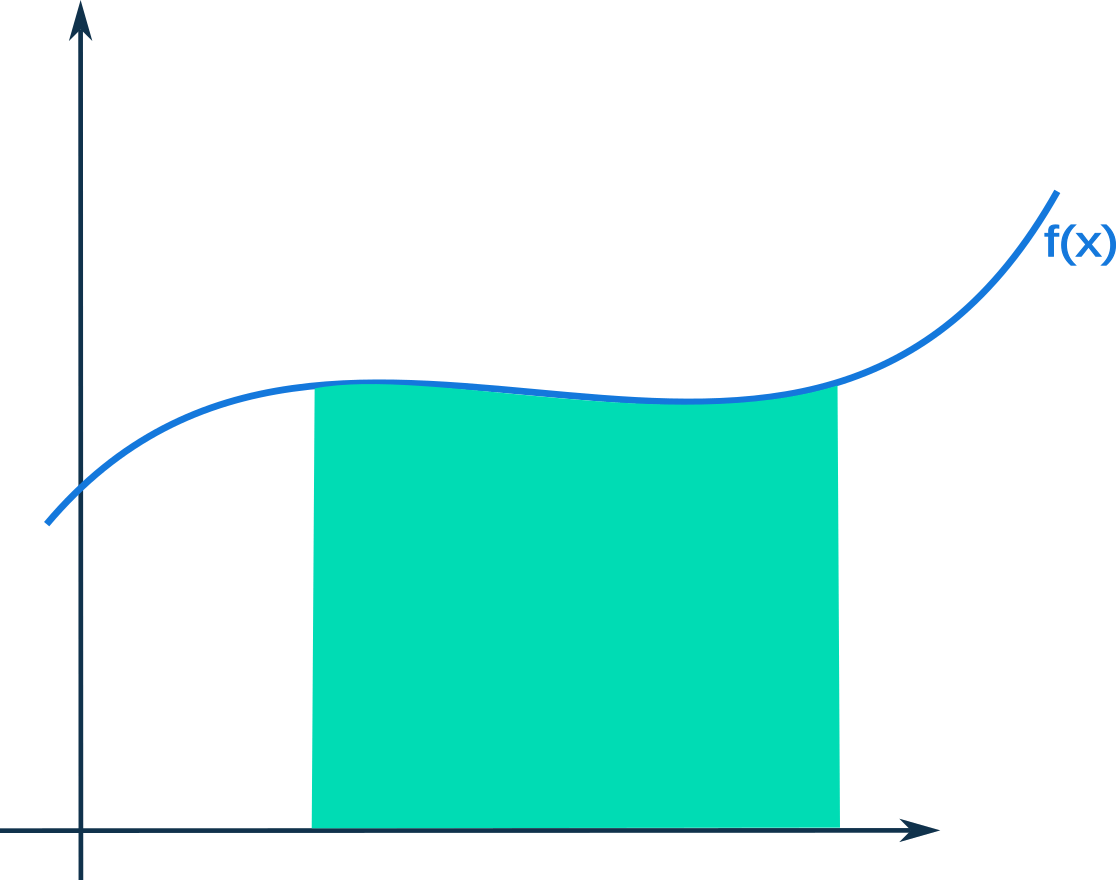Fig. 2. Area below the curve.
.
Definite Integrals are closely related to the area below a curve, so in this article we will explore how to evaluate definite integrals.
For more information about the area between curves and how to find it, see the article Area Between Two Curves.
Evaluating a Definite Integral Meaning and Example
To evaluate a definite integral means to find its value. This value is related to the area under the curve.
You might have noticed that an indefinite integral just has the integral symbol, \( \int,\) and after evaluating it you still have variables and an integration constant, for example\[\int x^2 \mathrm{d}x = \frac{1}{3}x^3+C.\]Definite integrals, instead, have integration limits, like \( \int_1^3,\) the end result is a number, and there are no integration constants, like
\[ \int_1^3 x^2 \mathrm{d}x = \frac{26}{3}\]
But how to evaluate a definite integral? There are many ways. The most common are:
By taking the limit of a Riemann Sum.
By substituting values using the Fundamental Theorem of Calculus.
From a graph by using a geometric formula.
Let's address each one at a time.
Evaluating a Definite Integral as a Limit
Begin by recalling the definition of a definite integral.
Let \( f(x) \) be a function defined in the interval \( [a,b]. \) Assuming the limit exists, the definite integral of \( f(x) \) from \( a \) to \( b \) is denoted as
\[ \int_a^b f(x)\,\mathrm{d}x, \]
and is defined as
\[ \int_a^b f(x)\,\mathrm{d}x=\lim_{N\rightarrow \infty} \sum_{i=1}^{N} f(x_i^*)\Delta x, \]
where
\[ \Delta x = \frac{b-a}{N}\]
and \(x_i^* \) is any point within a regular partition of the interval.
The values \( a \) and \( b \) are known as the limits of integration.
For more information and examples on setting up integrals, see the article Definite Integrals.
This means that a definite integral is defined as the limit of a Riemann sum as the number of subintervals tends to infinity. Please take a look at our Forming Riemann Sums article if you need a refresher on the subject!
Here is an example of how to evaluate a definite integral using limits.
Evaluate
\[ \int_{0}^{5} x^2\,\mathrm{d}x \]
using the definition of definite integral.
Solution:
In this case, the function is \( f(x)=x^2 \) and the limits of integration are \( a=0 \) and \( b=5.\) Knowing this, you can find \( \Delta x \) :
\[ \begin{align} \Delta x &= \frac{b-a}{N} \\ &= \frac{5-0}{N} \\ &= \frac{5}{N}. \end{align} \]
With this, you can use the definition of definite integral, so
\[ \int_0^5 x^2\,\mathrm{d}x = \lim_{N\rightarrow \infty} \left[ \sum_{i=1}^{N}\left( x_i^* \right)^2 \left( \frac{5}{N}\right) \right] .\]
You can use any point within each subinterval to evaluate the Riemann sum. For illustrative purposes, a right-endpoint approximation will be used. This will let you write
\[ \begin{align} x_i^* &= a+i\Delta x \\ &= i\frac{5}{N}, \end{align} \]
which can be substituted back into the definite integral
\[ \int_0^5 x^2\,\mathrm{d}x = \lim_{N\rightarrow \infty} \left[ \sum_{i=1}^{N}\left( i\frac{5}{N} \right)^2 \left( \frac{5}{N}\right) \right] .\]
To evaluate the above limit begin by rewriting the expression to simplify it a little:
\[\begin{align} \int_0^5 x^2\,\mathrm{d}x &= \lim_{N\rightarrow \infty} \left[ \sum_{i=1}^{N}\left( i\frac{5}{N} \right)^2 \left( \frac{5}{N}\right) \right] \\ &= \lim_{N\rightarrow \infty} \left[ \left( \frac{5}{N}\right)^3 \sum_{i=1}^{N}i^2 \right]. \end{align}\]
You can use the formula
\[\sum_{i=1}^{N}i^2=\frac{N(N+1)(2N+1)}{6}\]
to evaluate the sum, so
\[ \int_0^5 x^2 \,\mathrm{d}x = \lim_{N\rightarrow \infty} \left[ \left(\frac{5}{N}\right)^3 \left(\frac{N(N+1)(2N+1)}{6}\right) \right]. \]
The above expression can be rewritten by expanding the product, that is
\[ \int_0^5 x^2\,\mathrm{d}x = \lim_{N\rightarrow \infty} \left( \frac{125}{3}+\frac{125}{2N}+\frac{125}{6N^2} \right). \]
Finally, evaluate the above limit to find
\[ \int_0^5 x^2 \, \mathrm{d}x = \frac{125}{3}.\]
This result means that the area below \(f(x)=x^2\) in the interval \( [0,5] \) is equal to \( \frac{125}{3}.\)
You might have noticed that the above method is not the most practical one. Luckily, there is an easier way.
Evaluating a Definite Integral by substituting values
Another way of evaluating definite integrals is to use the evaluation part of the Fundamental Theorem of Calculus.
Let \(f(x)\) be a function that is integrable on the interval \( [a,b], \) and let \( F(x) \) be an antiderivative of \(f(x).\) The evaluation part of the Fundamental Theorem of Calculus states that
\[ \int_a^b f(x)\,\mathrm{d}x = F(b)-F(a).\]
This way you only need to find the antiderivative of the function and substitute some values.
Evaluate
\[\int_0^5 x^2 \, \mathrm{d}x \]
using the Fundamental Theorem of Calculus.
Answer:
Begin by finding the antiderivative of \(x^2.\) This can be done using the Power Rule, so
\[\int x^2 \, \mathrm{d}x = \frac{1}{3}x^3+C.\]
Next, you need to evaluate this antiderivative at both integration limits and subtract them. No matter what value of \(C\) you choose, it will cancel out when doing the subtraction, so there is no need to include it when using the Fundamental Theorem of Calculus. That means the integral is
\[ \begin{align} \int_0^5 x^2 \, \mathrm{d}x &= \left( \frac{1}{3}(5)^3 \right) - \left( \frac{1}{3}(0)^3 \right) \\ &= \frac{125}{3}. \end{align} \]
Note that you got the same answer using a more straightforward method!
Evaluating Definite Integrals Using Geometric Formulas
So far you have been using the definite integrals to find the area below a curve.
Let \(f(x)\) be a function that is non-negative and integrable on the interval \( [a,b].\) The area below the curve is given by its definite integral
\[A=\int_a^b f(x)\,\mathrm{d}x.\]
Some curves relate perfectly to geometric figures, so you can do the other way around! You can use the formulas for finding the area of geometric figures to find the value of definite integrals!
Evaluate the definite integral
\[ \int_0^4 2x\,\mathrm{d}x.\]
Answer:
In this case you are trying to find the area below the linear function \( f(x)=2x.\) Begin by taking a look at its graph.
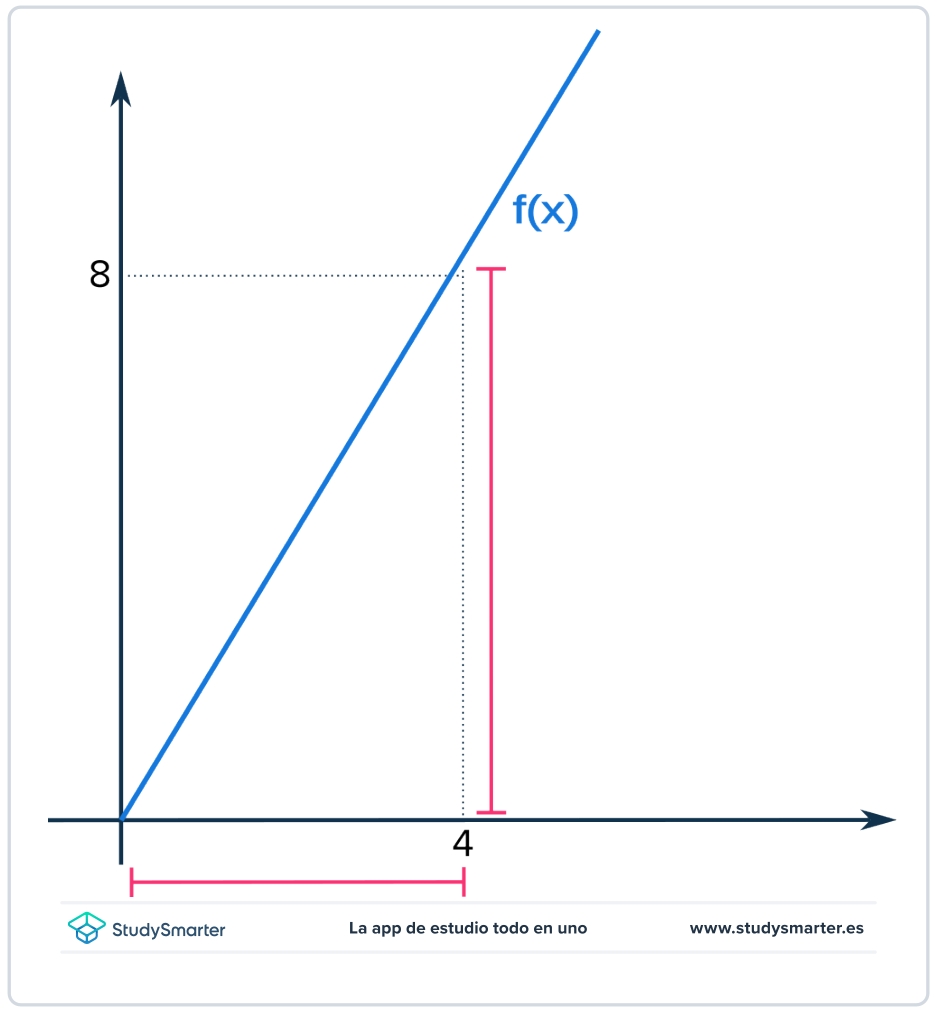Fig. 3. Graph of the linear function.
Note that the area below the function is a triangle with base 4 and height 8.
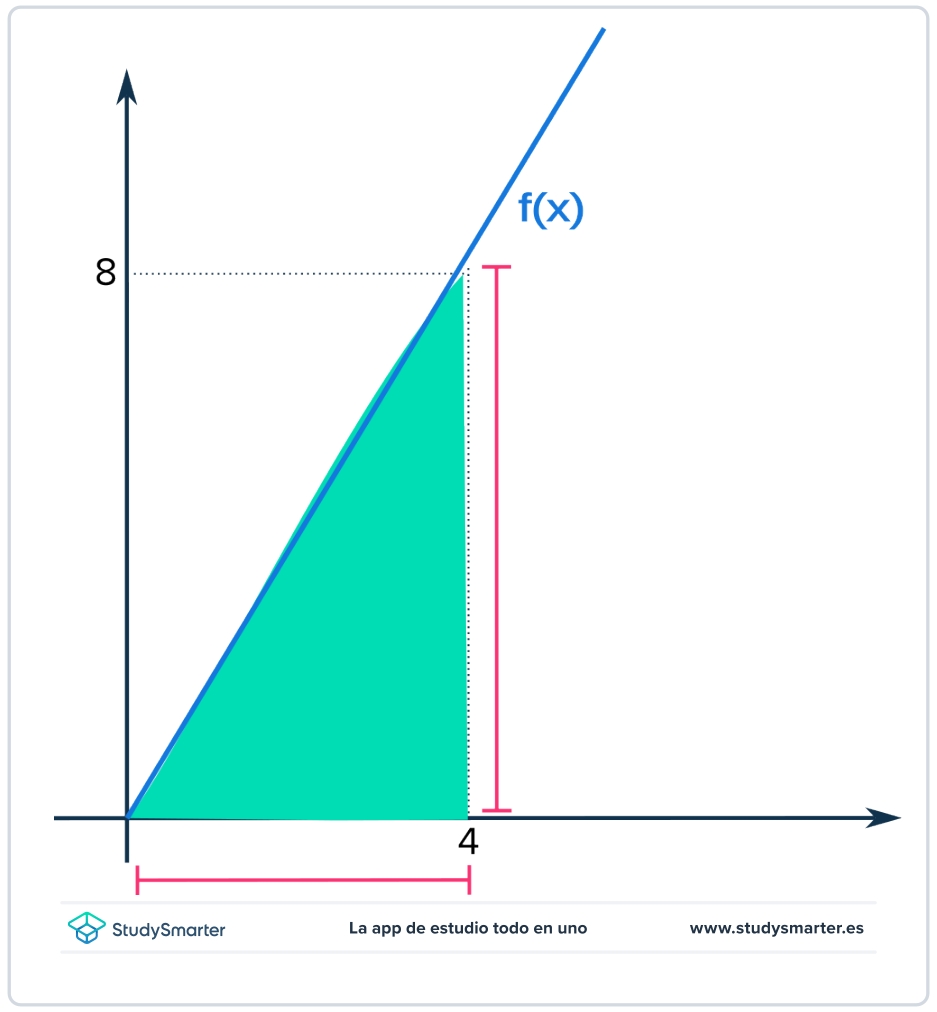Fig. 4. The area below the function forms a right triangle.
Therefore, you can use the formula for the area of a triangle to find this area, so
\[ \begin{align} A &= \frac{bh}{2} \\ &= \frac{4(8)}{2} \\ &= 16. \end{align} \]
This means that the value of the definite integral is 16.
\[ \int_0^4 2x \,\mathrm{d}x = 16 \]
This was much easier than finding the definite integral through its definition!
Take a look at another example.
Evaluate
\[\int_{-3}^3 \sqrt{9-x^2}\,\mathrm{d}x.\]
Answer:
You are looking for the area below the function \( f(x)=\sqrt{9-x^2}.\) By letting \(y=f(x),\) you can write an equation and cancel the square root by squaring both sides, that is
\[ \begin{align} y &= \sqrt{9-x^2} \\ y^2 &=9-x^2, \end{align}\]
from where you can obtain the equation of the standard form of a circumference,
\[x^2+y^2=9.\]
Please note that this function is only the top half of the circle, as a whole circle would fail to be a function!
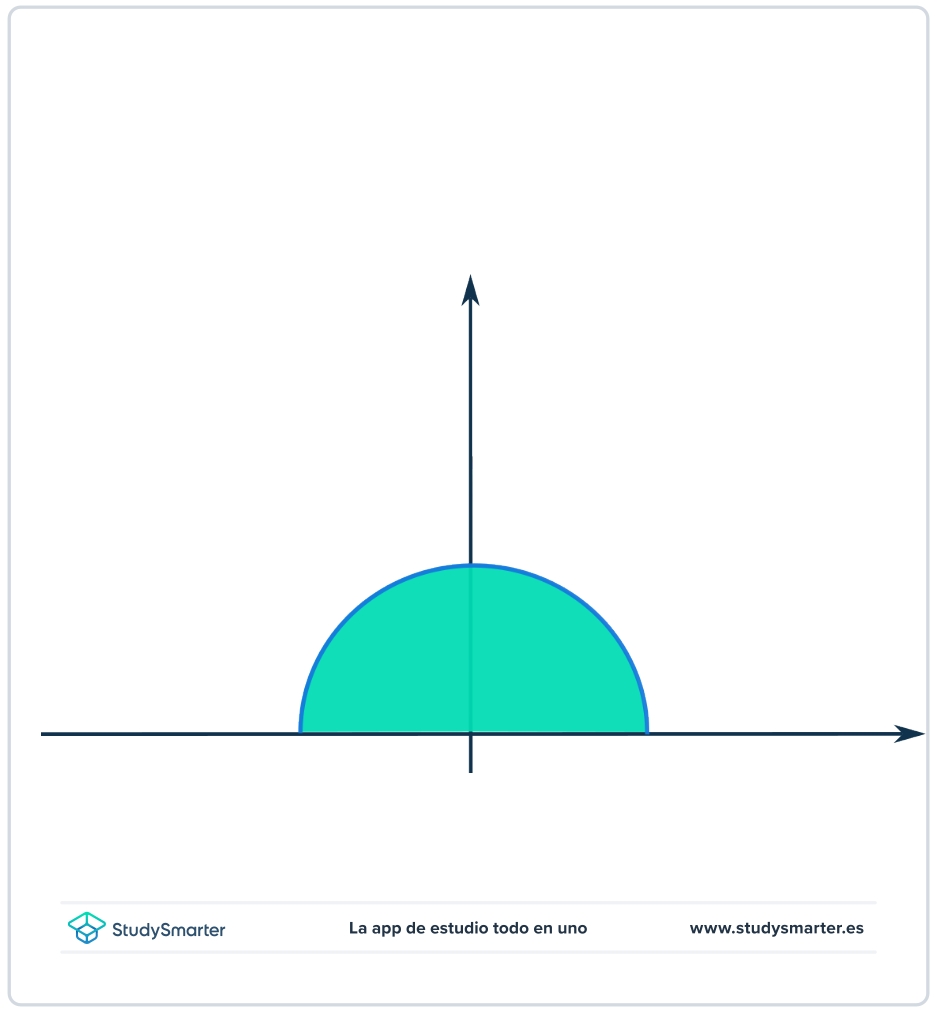Fig. 5. The given function draws the upper part of a circle.
The function that gives the bottom half of the circle would be \( f(x)= -\sqrt{9-x^2}.\)
The radius of this circle is 3, so you can find its area by using the area of a circle formula, that is
\[\begin{align} A_C &= \pi r^2 \\ &= \pi(3)^2 \\ &= 9\pi, \end{align} \]
but since the area below the curve is half the area of the circle, you need to take half of this result. Therefore
\[\int_{-3}^3\sqrt{9-x^2} \, \mathrm{d}x = \frac{9}{2}\pi. \]
Evaluating Definite Integrals from a graph
In general, functions have intervals where they are positive, and intervals where they are negative. What happens to the area of a function if its graph is below the x-axis? You can still assign it a value! However, areas cannot naturally be negative. In order to get over this, a convention is made by defining the signed area.
The signed area of a graph is such that:
- If the graph is above the x-axis, the area is defined as positive.
- If the graph is below the x-axis, the area is defined as negative.
A definite integral that involves these two types of intervals also has an area associated to it! You can find it by subtracting the area below the x-axis from the area above the x-axis.
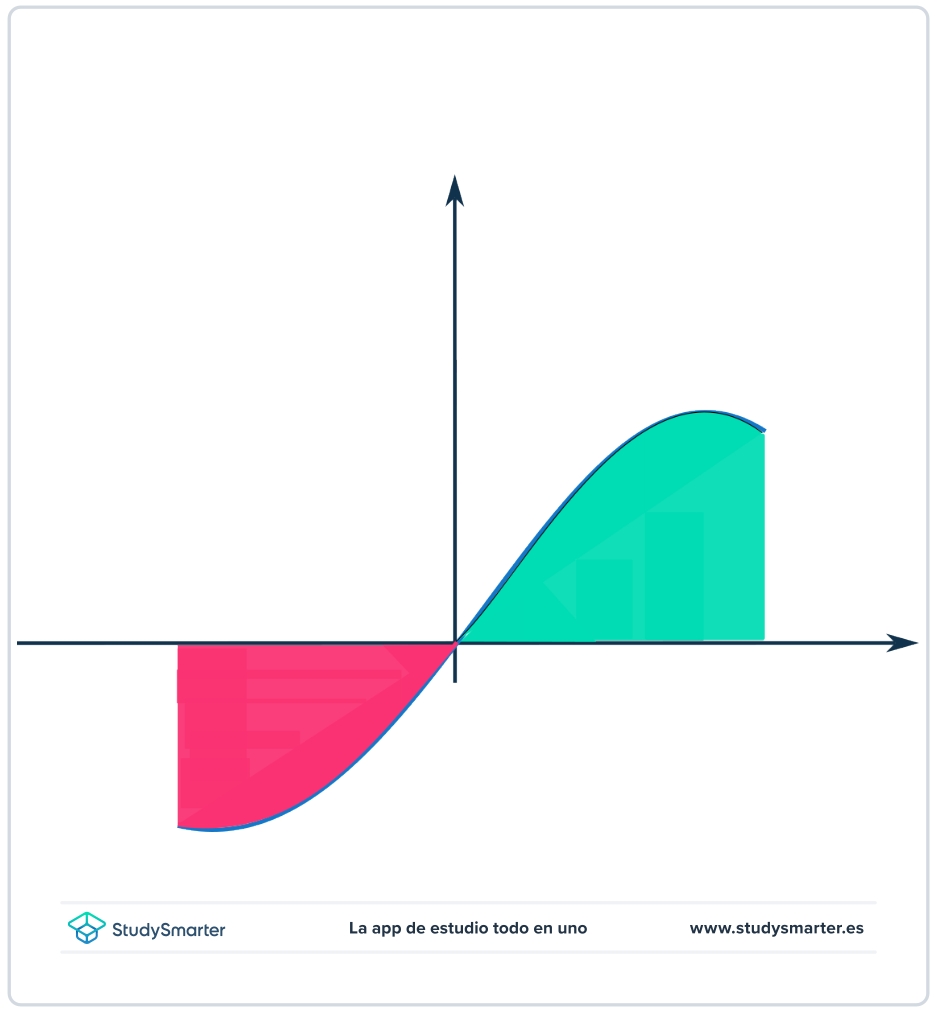Fig. 6. The area between the x-axis and a function with positive and negative intervals.
You can use a method of your choice to find the area of each portion of the graph.
Evaluating Definite Integrals - Key takeaways
- The definite integral \(\int_a^b f(x)\,\mathrm{d}x \) of an integrable and non-negative function gives you the area between \( f(x)\) and the x-axis.
- If the function is negative the area is defined negative.
- You can evaluate a definite integral by taking the limit of a Riemann sum as the number of subintervals tends to infinity.
- The evaluation part of the Fundamental Theorem of Calculus is a more practical way of evaluating definite integrals.
- If the area below a curve corresponds to a geometric figure you can use geometric formulas to evaluate the definite integral.
Explanations 
Exams 
Magazine 
