Explanations 
Flashcards 
StudySmarter AI 
Notes 
Study Plans 
Study Sets 
Exams 
Magazine 
Find a job 
Mobile App 
Jump to a key chapter
The rate at which this rumor spreads can be modeled with logistic growth! At first, the rumor spreads slowly as only a few people have heard it. However, as more students hear the rumor, the rate at which the rumor spreads increases. As the number of students who know the rumor begins to reach the total student population of \(1,000\), the rate at which the rumor spreads decreases as there are fewer students left to tell. Once all \(1,000\) students have heard the rumor, the rumor cannot spread any more. These are the basic elements of the logistic differential equation!
Logistic differential equation meaning
The logistic differential equation is used to model population growth that is proportional to the population's size and considers that there are a limited number of resources necessary for survival. The logistic differential growth model describes a situation that will stop growing once it reaches a carrying capacity. Essentially, the population cannot grow past a certain size as there are not enough life-sustaining resources to support the population.
Logistic differential equation formula
For a constant of proportionality \(k\), a population size \(P\), and some carrying capacity \(M\), the logistic differential equation is
\[\frac{dP}{dt}=kP\left(1-\frac{P}{M}\right)\]
and measures the growth of a population over time.
Logistic differential equation graph
The graph of the logistic equation is pictured below.
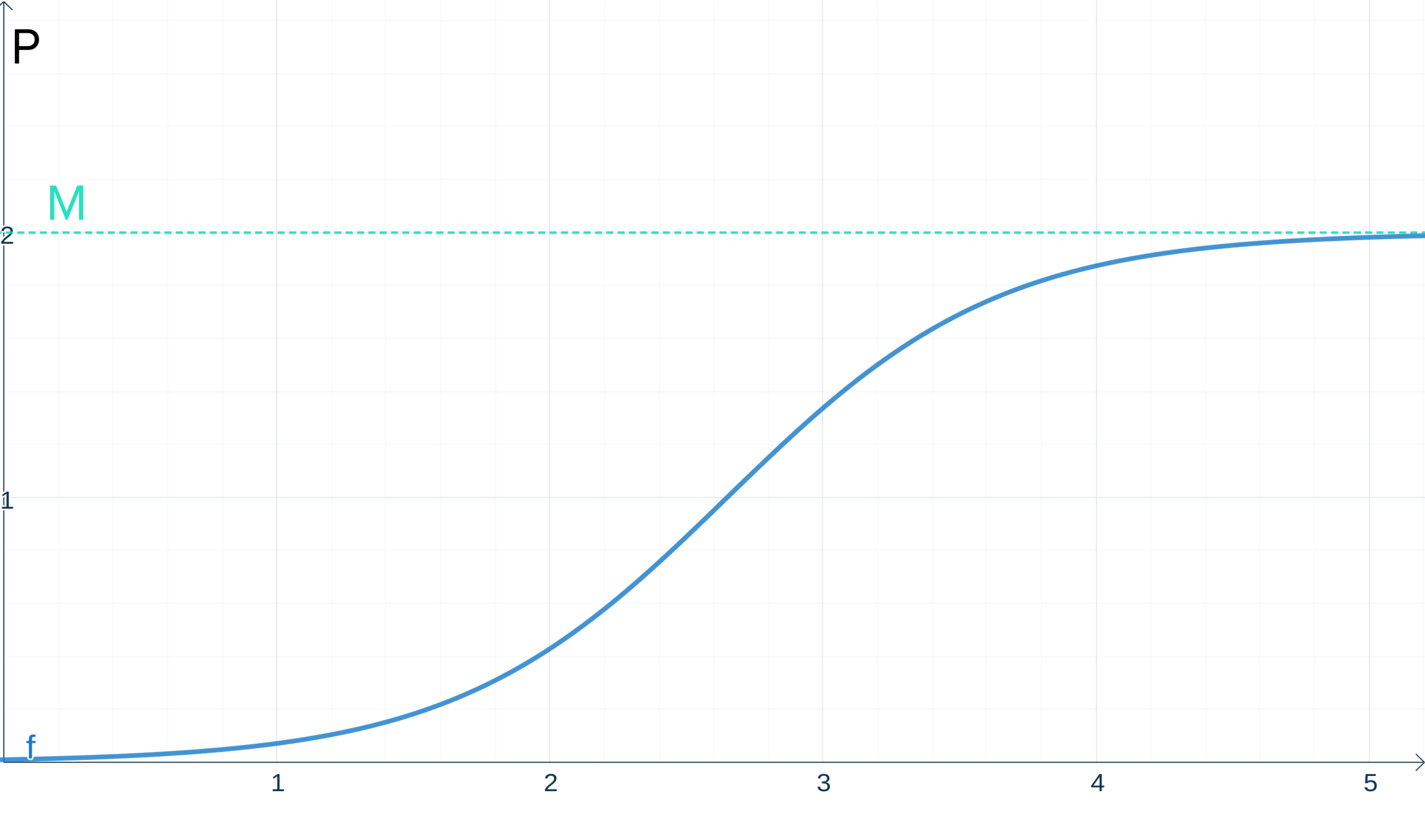Fig. 1. Graph of a logistic equation.
There is a point in the middle of the graph where the graph switches concavity. This is the point that the population growth rate begins to slow down. At first, the growth rate of the logistic growth model is almost identical to the exponential growth model. However, as time passes, the rate of growth begins to decrease. In other words, the population grows slower and slower as time passes until it reaches its carrying capacity \(M\). Notice how the graph does not exceed the carrying capacity.
Logistic differential equation limits and solutions
All solutions to the logistic differential equation are of the form
\[P(t)=\frac{M}{1+Ae^{-kt}}\]
where \(A\) is some constant that depends on the initial condition.
For the derivation of the logistic differential equation solution, see the Deep Dive below.
To solve the logistic differential equation, we will integrate it with separation of variables.
\[\int \frac{dP}{P(1- \frac{P}{M})}=\int k dt\]
Let's start with the left-hand side. First, we'll simply rewrite the fraction.
\[\int \frac{M}{P(M-P)}dP=\int\left(\frac{1}{P}+\frac{1}{M-P}\right)dP\]
Subbing back into the original equation
\[\int\left(\frac{1}{P}+\frac{1}{M-P}\right)dP=\int k dt\]
\[\int \frac{dP}{P} + \int \frac{dP}{M-P}= \int kdt\]
\[ln|P| - ln|M-P|=kt+C\]
\[ln\left|\frac{P}{M-P}\right|=kt+C\]
\[\frac{P}{M-P}=e^{kt+C}\]
\[\frac{P}{M-P}=Ae^{kt}\]
\[P=\frac{MAe^{kt} }{1+Ae^{kt}}\]
\[P=\frac{M}{\frac{1+Ae^{kt} }{Ae^{kt}}}\]
\[P(t)=\frac{M}{1+Ae^{-kt}}\]
With a basic knowledge of limits, we can see that no matter what the constant \(A\) is, \(\lim_{t \to \infty} P(t)=M\) as long as \(M\) and \(k\) are positive. Put into words, as long as the carrying capacity and the constant of proportionality are positive, regardless of the size of the population at \(t=0\), the population will reach the carrying capacity as time passes. Thus, the carrying capacity \(M\) can be thought of as an equilibrium value for the logistic model.
Logistic differential equation examples
Find the solution to the initial value problem \(\frac{dP}{dt}=0.08P(1- \frac{P}{1000})\) where \(P(0)=200\). Use the solution to find the population size at at \(t=20\) and \(t=100\).
Since we already know the general solution for a logistic differential equation, all we need to do is extract the pertinent information from the differential equation and plug it into the solution.
Referencing the general logistic differential equation, we can see that the proportionality constant \(k\) equals 0.08 and the carrying capacity \(M\) equals 1000. Let's go ahead and plug these values into the logistic differential general solution.
\[P(t)=\frac{1000}{1+Ae^{-0.08t}}\]
All we're missing is the constant \(A\). Luckily, we can use the initial value from the problem to solve for \(A\)!
\[P(0)=\frac{1000}{1+Ae^{-0.08(0)}}\]
\[200=\frac{1000}{1+Ae^{0}}\]
\[200+200A=1000\]
\[200+200A=1000\]
\[A=4\]
So, we have a solution to \(\frac{dP}{dt}\):
\[P(t)=\frac{1000}{1+4e^{-0.08t}}\]
We can use this solution to find the population size at \(t=20\) and \(t=100\) by simply plugging in, solving, and rounding to the nearest whole number.
\[P(20)=\frac{1000}{1+4e^{-0.08(20)}}\]
\[P(20)\approx 553\]
\[P(100)=\frac{1000}{1+4e^{-0.08(100)}}\]
\[P(100)=999\]
A population \(P\) has a rate of change of \(\frac{dP}{dt}=0.3P(5000-P)\). When is the population size increasing at an increasing rate? When is the population size increasing at a decreasing rate?
The population size is growing when \(\frac{dP}{dt}>0\). To check when the population size is increasing at an increasing rate, we need to look for when \(\frac{d^{2}P}{dt^{2}}>0\). So, let's find out when both the first and second derivatives are greater than 0.
First, we'll set the first derivative equal to 0.
\[\frac{dP}{dt}=0.3P(5000-P)=0\]
\[1500P-0.3P^{2}=0\]
\[P(1500-0.3P)=0\]
\[P=0\; or\; P=5000\]
When \(0 \le P < 5000\), \(\frac{dP}{dt}>0\). So, \(P\) is increasing between 0 and 5,000.
Now, we'll find the second derivative and set it equal to 0.
\[\frac{d^{2}P}{dt^{2}}=1500-0.6P\]
\[\frac{d^{2}P}{dt^{2}}=1500-0.6P=0\]
\[1500-0.6P=0\]
\[P=2500\]
When \(P<2500\), \(\frac{d^{2}P}{dt^{2}}>0\). So, the population size is increasing at an increasing rate when the population is between 0 and 2,500.
When \(P>2500\), \(\frac{d^{2}P}{dt^{2}}<0\). So, the population size is increasing at a decreasing rate when the population is between 2,500 and 5,000.
Referencing the graph of the logistic equation pictured at the beginning article, the graph changes concavity when the population is at 2,500. Essentially, when the population reaches 2,500 people, the rate at which the population grows slows down.
Logistic Differential Equation - Key takeaways
- The logistic differential equation is used to model population growth that is proportional to the size of the population and takes into account that there are a limited number of resources necessary for survival - it describes a situation where the population will stop growing once it reaches a carrying capacity
- For a constant of proportionality \(k\), a population size \(P\), and some carrying capacity \(M\), the logistic differential equation is \(\frac{dP}{dt}=kP(1-\frac{P}{M})\) and measures the growth of a population over time
- All solutions to the logistic differential equation are of the form \(P(t)=\frac{M}{1+Ae^{-kt}}\) where \(A\) is some constant that depends on the initial condition
- No matter what the constant \(A\) is, \(\lim_{t \to \infty} P(t)=M\) as long as \(M\) and \(k\) are positive; thus \(M\) can be thought of as an equilibrium value
Learn faster with the 2 flashcards about Logistic Differential Equation
Sign up for free to gain access to all our flashcards.
Frequently Asked Questions about Logistic Differential Equation
What is the logistic differential equation?
The logistic differential equation is used to model population growth that is proportional to the size of the population and takes into account that there are a limited number of resources necessary for survival. The logistic differential growth model describes a situation where population will stop growing once it reaches a carrying capacity. Essentially, the population cannot grow past a certain size as there are not enough life sustaining resources to support the population.
How do you solve logistics differential equation?
The logistic differential equation has a general solution P(t). Every solution to the logistic differential equation follows the form of this solution.
What is the formula for logistics differential equation?
The logistic differential equation formula is dP/dt = kP(1 - (P/M)) where k is a constant of proportionality, P is the population size, and M is some carrying capacity.
How is logistics differential equation derived?
The logistic differential equation is derived by taking the derivative of the logistic equation.
How do you graph logistic differential equation?
The logistic differential equation can be graphed as a slope field. Or, if provided with an initial condition, it can be graphed as a logistic sigmoid curve.
About StudySmarter
StudySmarter is a globally recognized educational technology company, offering a holistic learning platform designed for students of all ages and educational levels. Our platform provides learning support for a wide range of subjects, including STEM, Social Sciences, and Languages and also helps students to successfully master various tests and exams worldwide, such as GCSE, A Level, SAT, ACT, Abitur, and more. We offer an extensive library of learning materials, including interactive flashcards, comprehensive textbook solutions, and detailed explanations. The cutting-edge technology and tools we provide help students create their own learning materials. StudySmarter’s content is not only expert-verified but also regularly updated to ensure accuracy and relevance.
Learn more