Manipulating Functions

Manipulating Functions: Algebra - A Quick Review

While functions are at the heart of calculus, algebra, and therefore algebraic manipulation, is the language of calculus. We can’t do calculus without algebra! So, if your memory is the least bit foggy on the rules for fractions, absolute values, powers, roots, logs, factoring, and solving quadratic equations, this is where we review those.

Manipulating Fractions

Fractions are everywhere in calculus. No matter how much we want to, we can’t escape them. Here are some rules for, properties of, and techniques to use with fractions that we need to remember:

• Rules:

  • Never divide by zero! If the denominator of a fraction is zero, then it is undefined.

  • BUT, the numerator of a fraction can be zero.

    • Any fraction with a zero in the numerator is equal to zero, as long as zero is not in the denominator.

  • When adding or subtracting fractions, the denominators must be equal.

    • To add or subtract fractions, the Least Common Denominator (LCD) must be found.

  • If the denominator of a fraction is 1, then the fraction simplifies to an integer.

  • If the numerator and denominator of a fraction are equal, then the fraction simplifies to 1.

  • Rule of equivalent fractions:

    • Two fractions $\raisebox{1ex}{$a$}\!\left/ \!\raisebox{-1ex}{$b$}\right.$ and $\raisebox{1ex}{$c$}\!\left/ \!\raisebox{-1ex}{$d$}\right.$ are equal and can be written as $\raisebox{1ex}{$a$}\!\left/ \!\raisebox{-1ex}{$b$}\right.=\raisebox{1ex}{$c$}\!\left/ \!\raisebox{-1ex}{$d$}\right.$ if $a\times d=b\times c$

  • Remember the reciprocal. If we flip a fraction upside down, we get its reciprocal.

    • Multiplying a fraction by its reciprocal always gives us 1, provided the numerator of the original fraction is not zero.

• Properties:

  • Commutativity of addition with fractions:

    • $\frac{a}{b}+\frac{c}{d}=\frac{c}{d}+\frac{a}{b}$

  • Commutativity of multiplication with fractions:

    • $\frac{a}{b}\times \frac{c}{d}=\frac{c}{d}\times \frac{a}{b}$

  • Associativity of addition with fractions:

    • $\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left(\frac{a}{b}+\frac{c}{d}\right)+\frac{e}{f}=\frac{a}{b}+\left(\frac{c}{d}+\frac{e}{f}\right)$

  • Associativity of multiplication with fractions:

    • $\frac{a}{b}\times \frac{c}{d}\times \frac{e}{f}=\left(\frac{a}{b}\times \frac{c}{d}\right)\times \frac{e}{f}=\frac{a}{b}\times \left(\frac{c}{d}\times \frac{e}{f}\right)$

  • Distributivity of multiplication over addition with fractions:

    • $\frac{a}{b}\left(\frac{c}{d}+\frac{e}{f}\right)=\left(\frac{a}{b}\times \frac{c}{d}\right)+\left(\frac{a}{b}\times \frac{e}{f}\right)$

• Techniques:

  • We can use multiplication or division to make equivalent fractions:

    • $\frac{a}{b}=\frac{a\times c}{b\times c},wherec\ne 0$

    • $\frac{a}{b}=\frac{a÷c}{b÷c},wherec\ne 0$

  • We can write integers as fractions:

    • $a=\frac{a\times c}{c},wherec\ne 0$

  • Adding fractions:

    • $\frac{a}{b}+\frac{c}{b}=\frac{a+c}{b}$, for fractions with the same denominator

    • $\frac{a}{c}+\frac{b}{d}=\frac{a}{c}\times \frac{d}{d}+\frac{b}{d}\times \frac{c}{c}=\frac{a\times d}{c\times d}+\frac{b\times c}{d\times c}=\frac{a\times d+b\times c}{c\times d}$, for fractions with different denominators

  • Subtracting fractions:

    • $\frac{a}{b}-\frac{c}{b}=\frac{a-c}{b}$, for fractions with the same denominator

    • $\frac{a}{c}-\frac{b}{d}=\frac{a}{c}\times \frac{d}{d}-\frac{b}{d}\times \frac{c}{c}=\frac{a\times d}{c\times d}-\frac{b\times c}{d\times c}=\frac{a\times d-b\times c}{c\times d}$, for fractions with different denominators

  • Multiplying fractions:

    • $\frac{a}{b}\times \frac{c}{d}=\frac{a\times c}{b\times d}$

  • Dividing fractions:

    • $\frac{a}{b}÷\frac{c}{d}=\frac{a}{b}\times \frac{d}{c}=\frac{a\times d}{b\times c}$

  • Simplifying (also called canceling) fractions:

    • It is important to remember that we can only cancel in a fraction when it has an unbroken chain of multiplication throughout the entire numerator and denominator.

    • $\frac{\cancel{ab}c}{\cancel{ab}d}$can be simplified to $\frac{c}{d}$, but $\frac{abc+d}{abd}$is in its simplest form (cannot cancel $a,b,ord$here).

Absolute Values

Taking the absolute value of a number means turning a negative number positive, and doing nothing to a positive number or zero. For example:

$\left|-4\right|=4$

$\left|4\right|=4$

$\left|0\right|=0$

The straight lines surrounding the number means “the absolute value of”. So when we see this notation, we know to take the absolute value of the number or variable inside.

Manipulating Powers

The rules of powers (also known as exponents) come in very handy in our study of AP Calculus. They make solving problems much easier. And don’t forget, these rules work in reverse, too! But first, let's recap a couple of definitions.

Now, let's recap the power rules. For these, we need to make a couple of assumptions:

1. We assume X and Y are nonzero real numbers.

2. We assume that m and n are integers.

The power rules are listed as follows:

• Product rule: $x^m{x}^n=x^{m+n}$

• Quotient rule: $x^m÷x^n=\frac{x^m}{x^n}=x^{m-n}$

• Negative exponent rule: $x^{-m}=\frac{1}{x^m}$

• Zero power rule: $x^0=1,wherex\ne 0$

• Power of a power rule: ${\left(x^m\right)}^n=x^{m\times n}$

• Product to a power rule: $x^n{y}^n={\left(xy\right)}^n$

• Quotient to a power rule: $\frac{x^n}{y^n}={\left(\frac{x}{y}\right)}^n$

• Fractional power rule: $x^{\frac{m}{n}}=\sqrt[n]{x^m}={\left(\sqrt[n]{x}\right)}^m$

Manipulating Roots

As you might have noticed from the power rules, powers and roots are related. This relationship is extremely useful when solving and simplifying AP Calculus problems. Basically, any root can be converted to a power, and vice versa. The root rules are summarized below.

• The number under an even root (2, 4, 6, etc.) can’t be negative!

• But the number under an odd root (3, 5, 7, etc.) can be negative.

• For roots where n is an even number: $\sqrt[n]{x^n}=\left|x\right|$

• For roots where n is an odd number: $\sqrt[n]{x^n}=x$

• Zero root rule: $\sqrt{0}=0$

• Identity root rule: $\sqrt{1}=1$

• Product rule: $\left(\sqrt[n]{x}\right)\left(\sqrt[n]{y}\right)=\sqrt[n]{xy}$

• Quotient rule: $\frac{\sqrt[n]{x}}{\sqrt[n]{y}}=\sqrt[n]{\frac{x}{y}}$

• Root to a root rule: $\sqrt[m]{\sqrt[n]{x}}=\sqrt[m\times n]{x}$

• Rationalizing the denominator:

  • By convention, we don't want roots in the denominator of a fraction. We can remove them by a process called rationalization, where we multiply the fraction with a root in its denominator by another fraction (that otherwise would simplify to 1) with that same root in its denominator so that the root is canceled out. For example:

    • $\frac{1}{\sqrt{3}}=\frac{1}{\sqrt{3}}\times \frac{\sqrt{3}}{\sqrt{3}}=\frac{\sqrt{3}}{3}$

Manipulating Logarithms

A logarithmic expression is another way of writing an exponential expression. The base of a logarithmic expression can be any positive number, except for 1. If we want to use e as the base of a logarithmic expression, we write ln instead of log. If we want to use 10 as the base of a logarithmic expression, by convention, we don’t write it. The rules for logarithmic expressions are summarized below.

• Zero log rule: ${\log }_{n}1=0,wheren>0butn\ne 1$

• Identity log rule: ${\log }_{n}n=1$

• Product rule: ${\log }_{n}xy={\log }_{n}x+{\log }_{n}y$

• Quotient rule: ${\log }_n\frac{x}{y}={\log }_{n}x-{\log }_{n}y$

• Power rule: ${\log }_n{x}^m=m\left({\log }_{n}x\right)$

• Log of a power rule: ${\log }_n{n}^m=m$

• Power of a log rule: $n^{{\log }_{n}m}=m$

Factoring

Another super helpful tool for AP calculus is factoring. Factoring is like breaking a number into its prime factors, but for algebraic expressions. Factoring an expression involves rewriting a sum of terms as a product of terms. We do this by pulling out - or factoring - the Greatest Common Factor (GCF) from all the terms of the expression.

Once we pull out the GCF, the next step is to look for one of the following patterns:

• Difference of squares - it is absolutely necessary to know how to factor this!

  • $x^2-y^2=\left(x-y\right)\left(x+y\right)$

  • A difference of squares can be factored, but a sum of squares cannot.

• Sum of cubes

  • $x^3+y^3=\left(x+y\right)\left(x^2-xy+y^2\right)$

• Difference of cubes

  • $x^3-y^3=\left(x-y\right)\left(x^2+xy+y^2\right)$

• Square of the sum of two numbers

  • ${\left(x+y\right)}^2=x^2+2xy+y^2$

• Square of the difference of two numbers

  • ${\left(x-y\right)}^2=x^2-2xy+y^2$

• Cube of the sum of two numbers

  • ${\left(x+y\right)}^3=x^3+3x^{2}y+3x{y}^2+y^3$

• Cube of the difference of two numbers

  • ${\left(x-y\right)}^3=x^3-3x^{2}y+3x{y}^2-y^3$

• Square of the sum of three numbers

  • ${\left(x+y+z\right)}^2=x^2+y^2+z^2+2xy+2xz+2yz$

• Square of the difference of three numbers

  • ${\left(x-y-z\right)}^2=x^2+y^2+z^2-2xy-2xz+2yz$

Solving Quadratic Equations

Going back to algebra days, a quadratic equation is a second-degree polynomial. Quadratic equations can be solved by one of the following methods.

Factoring a Quadratic Equation

If it is possible, factoring a quadratic equation is perhaps the easiest and quickest way to solve a quadratic equation. We can test if a quadratic equation is factorable by finding its discriminant. If the discriminant is a perfect square, then the quadratic equation is factorable.

The Quadratic Formula

Regardless if a quadratic equation is factorable, we can always solve it using the quadratic formula. For a quadratic equation of the form:

$a{x}^2+bx+c=0$

We can find the solutions using the quadratic formula:

$x=\frac{-b\pm \sqrt{b^2-4ac}}{2a}$

Completing the Square

This method for solving a quadratic equation is aptly named because it involves taking the quadratic formula in question and making it a perfect square so that it is solvable by taking the square root. We can always use this method to solve a quadratic equation as well, regardless of if it is factorable.

Manipulating Functions: A Refresher on Trig Functions

Many of the problems we will encounter in AP Calculus involve manipulating trig functions. So, for those of us who don't want to have to relearn trigonometry at the same time as learning AP Calculus, this refresher is key.

Trigonometry starts with triangles - specifically right triangles. The image and table below summarizes key terms of right triangles and the six main trig functions.

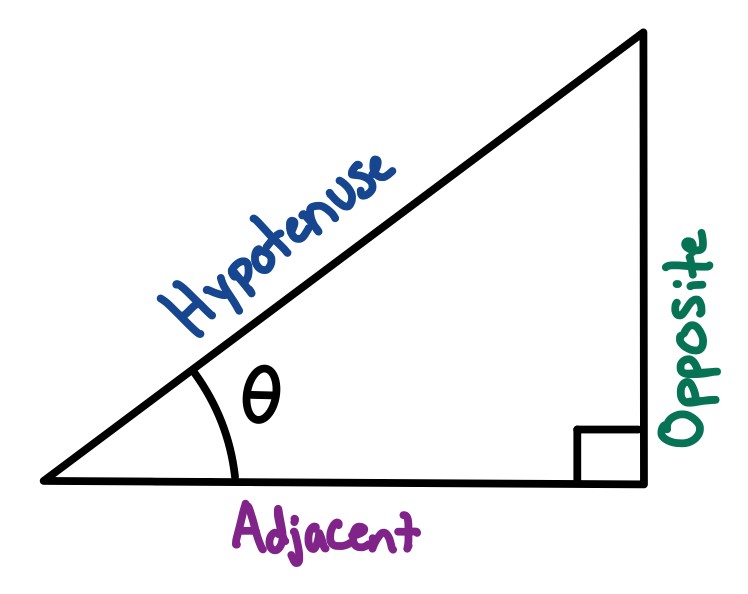A right triangle with its sides labeled - StudySmarter Originals

A way to remember Sin, Cos, and Tan ratios

The main trig functions are defined by ratios of the sides of a right triangle in a specific order. The side labeled Hypotenuse (or just H for short) is always the triangle's longest side. The side labeled Adjacent (or just A for short) is the side that is touching the angle of the triangle we are considering (which is θ in the image above). The side labeled Opposite (or just O for short) is the side that is across from the angle. SohCahToa (pronounced "So-Kah-Toe-Ah") is a mnemonic device used to help us remember the ratios that make up the sine, cosine, and tangent trig functions.

From there, we can remember cosecant, secant, and cotangent as the reciprocals of sine, cosine, and tangent.

With that in mind, there are two special right triangles that we deal with in a lot of AP Calculus problems that deserve mention.

Special Right Triangles

Also called an isosceles right triangle, the 45-45-90 triangle is exactly what it sounds like: a right triangle whose other two angles are 45 degrees. What is special about this triangle is that the two legs of the triangle that touch the right angle are always the same size, and the hypotenuse is always $\sqrt{2}$ times longer than the two legs. It's a good idea to memorize the trig functions for this triangle.

$$\begin{gathered} sin\left(45°\right)=\frac{O}{H}=\frac{a}{\sqrt{2}\times a}=\frac{1}{\sqrt{2}}=\frac{1}{\sqrt{2}}\times \frac{\sqrt{2}}{\sqrt{2}}=\frac{\sqrt{2}}{2}csc\left(45°\right)=\frac{H}{O}=\frac{\sqrt{2}\times a}{a}=\sqrt{2} \\ cos\left(45°\right)=\frac{A}{H}=\frac{a}{\sqrt{2}\times a}=\frac{1}{\sqrt{2}}=\frac{1}{\sqrt{2}}\times \frac{\sqrt{2}}{\sqrt{2}}=\frac{\sqrt{2}}{2}sec\left(45°\right)=\frac{H}{A}=\frac{\sqrt{2}\times a}{a}=\sqrt{2} \\ tan\left(45°\right)=\frac{O}{A}=\frac{a}{a}=1cot\left(45°\right)=\frac{A}{O}=\frac{a}{a}=1 \end{gathered}$$

The 30-60-90 right triangle is also aptly named. What is special about this triangle is that the hypotenuse is always twice as long as the shortest leg, and the longer leg is always $\sqrt{3}$ times longer than the shorter leg. It's also good to memorize the trig functions for this triangle for both the $30°$ and $60°$angles.

$$\begin{gathered} sin\left(30°\right)=\frac{O}{H}=\frac{a}{2a}=\frac{1}{2}csc\left(30°\right)=\frac{H}{O}=\frac{2a}{a}=2 \\ cos\left(30°\right)=\frac{A}{H}=\frac{\sqrt{3}\times a}{2a}=\frac{\sqrt{3}}{2}sec\left(30°\right)=\frac{H}{A}=\frac{2a}{\sqrt{3}\times a}=\frac{2}{\sqrt{3}}\times \frac{\sqrt{3}}{\sqrt{3}}=\frac{2\sqrt{3}}{3} \\ tan\left(30°\right)=\frac{O}{A}=\frac{a}{\sqrt{3}\times a}=\frac{1}{\sqrt{3}}\times \frac{\sqrt{3}}{\sqrt{3}}=\frac{\sqrt{3}}{3}cot\left(30°\right)=\frac{A}{O}=\frac{\sqrt{3}\times a}{a}=\sqrt{3} \end{gathered}$$

$$\begin{gathered} sin\left(60°\right)=\frac{O}{H}=\frac{\sqrt{3}\times a}{2a}=\frac{\sqrt{3}}{2}csc\left(60°\right)=\frac{H}{O}=\frac{2a}{\sqrt{3}\times a}=\frac{2}{\sqrt{3}}\times \frac{\sqrt{3}}{\sqrt{3}}=\frac{2\sqrt{3}}{3} \\ cos\left(60°\right)=\frac{A}{H}=\frac{a}{2a}=\frac{1}{2}sec\left(60°\right)=\frac{H}{A}=\frac{2a}{a}=2 \\ tan\left(60°\right)=\frac{O}{A}=\frac{\sqrt{3}\times a}{a}=\sqrt{3}cot\left(60°\right)=\frac{A}{O}=\frac{a}{\sqrt{3}\times a}=\frac{1}{\sqrt{3}}\times \frac{\sqrt{3}}{\sqrt{3}}=\frac{\sqrt{3}}{3} \end{gathered}$$

A more convenient way to view the trig functions of these angles (plus angles of 0 and 90 degrees) is with a table.

The Unit Circle

While the SohCahToa mneumonic device is great for right triangles, it doesn't work for angles at or greater than 90 degrees. For those, we need the unit circle. The unit circle allows us to find trig values for any angle, not just acute ones. The unit circle has a radius of one unit (hence the name) and is set in the x-y coordinate plane.

The above image tells us quite a lot. So let's walk through it a bit. To start, when we measure angles using the unit circle, we start at the positive x-axis and rotate counterclockwise (see the $150°$ angle in the image). If we rotated clockwise, we would get a negative angle (see the $-70°$ angle in the image). This is true regardless of whether we are measuring the angle in degrees or radians. In AP Calculus, radians are almost always the preferred way to measure angles. Remember, the radian measure of an angle is the length of the arc along the circumference of the unit circle (see the bolded edge of the circle labeled $Length=\frac{\pi }{6}$). The easiest way to convert between degrees and radians is the formula:

$$\begin{gathered} \frac{\mathrm{\pi }}{180}=\frac{R}{\theta }\Rightarrow R=\frac{\mathrm{\pi \theta }}{180}and\theta =\frac{180R}{\mathrm{\pi }} \\ where, \\ \theta istheangleindegrees \\ Ristheangleinradians \end{gathered}$$

A closer look at the unit circle above reveals the two 30-60-90 right triangles. One is in the first quadrant of the circle, the other is in the second quadrant. Based on these two triangles, we can gather that the coordinates on the unit circle tell us the angle's cosine and sine values.

With this in mind, let's see the unit circle with all of the cosine and sine values plotted for the 30-60-90 and 45-45-90 triangles.

It is important to note that the values of all the main trig functions are positive in the first quadrant, only sine and cosecant are positive in the second quadrant, only tangent and cotangent are positive in the third quadrant, and only cosine and secant are positive in the fourth quadrant of the unit circle. Another helpful mnemonic device to remember which functions are positive in what quadrants is All Students Take Calculus.

• All - All 6 trig functions are positive in the first quadrant.

• Students - only Sine (and its reciprocal, cosecant) are positive in the second quadrant.

• Take - only Tangent (and its reciprocal, cotangent) are positive in the third quadrant.

• Calculus - only Cosine (and its reciprocal, secant) are positive in the fourth quadrant.

Graphing Trig Functions

The main trig functions - sine, cosine, and tangent - and their reciprocals - cosecant, secant, and cotangent - are periodic functions. This means their graphs are the same shape repeated over and over again, indefinitely. The period of these functions is the length of one of their cycles.

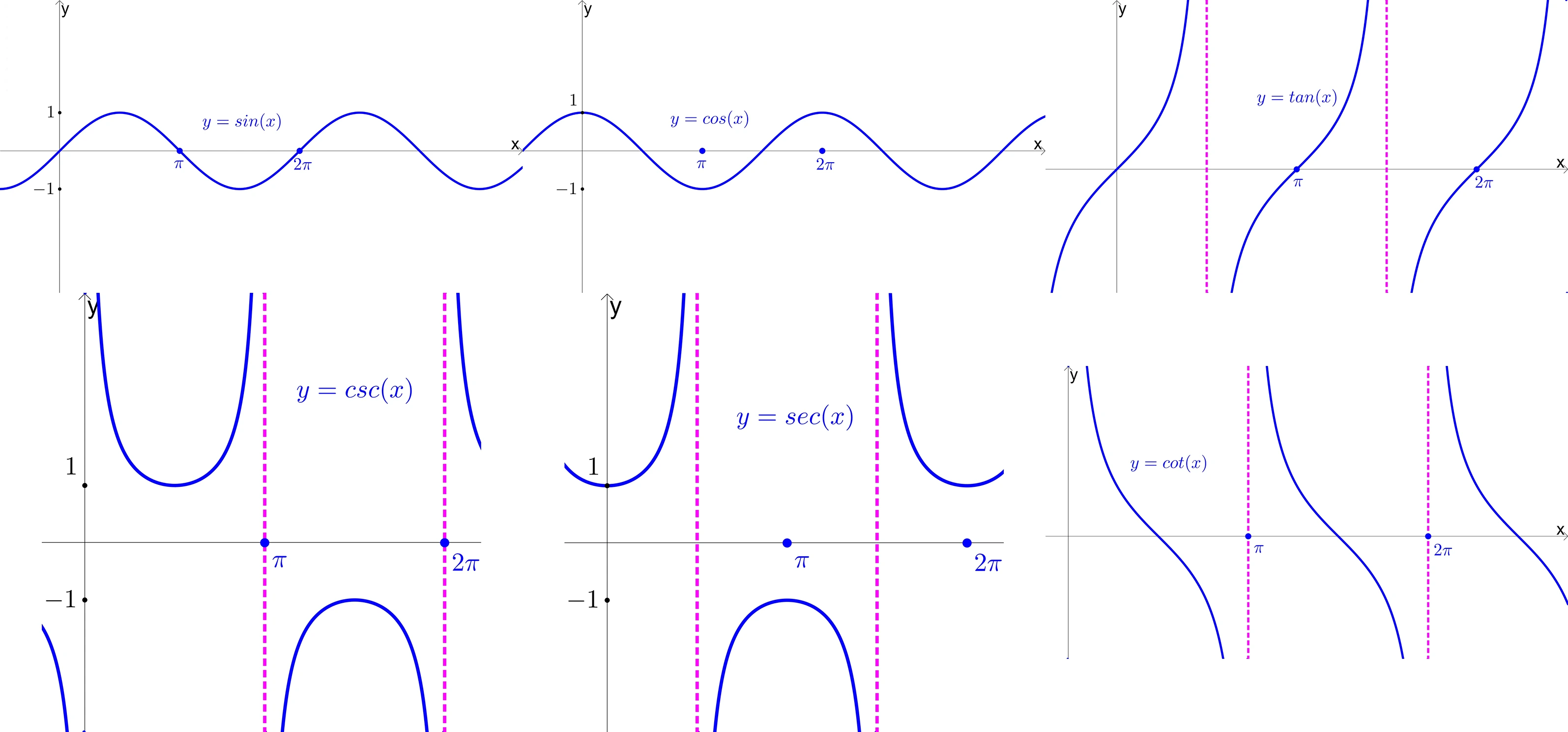The graphs of all 6 trig functions - StudySmarter Originals

Now that we have refreshed the unit circle, we can sketch the graphs of sine, cosine, and tangent easily by noting some important facts:

• For sine and cosine:

  • The graphs of sine and cosine are the same shape.

    • The cosine graph is just shifted to the left 90 degrees.

  • The sine and cosine graphs have a max of 1 and a min of -1.

  • The sine and cosine graphs repeat their shapes indefinitely to the left and right.

    • Their shapes repeat every 360 degrees, so their period is 360 degrees (which is the same number of degrees a circle has)

  • The unit circle tells us that:

    • $\sin \left(0°\right)=0and\cos \left(0°\right)=1$

    • $\sin \left(90°\right)=1and\cos \left(90°\right)=0$

    • $\sin \left(180°\right)=0and\cos \left(180°\right)=-1$

    • $\sin \left(270°\right)=-1and\cos \left(270°\right)=0$

    • $\sin \left(360°\right)=0and\cos \left(360°\right)=1$

  • Using these 5 points, we can sketch one cycle of the sine and cosine functions.

• For tangent:

  • The basic shape of the graph is a backward S

    • This shape is repeated indefinitely to the left and right.

      • It repeats every 180 degrees, so its period is 180 degrees.

  • Because $\tan \left(\theta \right)=\frac{\sin \left(\theta \right)}{\cos \left(\theta \right)}=\frac{y}{x}$, we can use the unit circle to sketch the graph:

    • $\tan \left(-45°\right)=-1$

    • $\tan \left(0°\right)=0$

    • $\tan \left(45°\right)=1$

    • Because $\tan \left(-90°\right)=undefinedand\tan \left(90°\right)=undefined$, we draw vertical asymptotes at those points.

Trig Identities and Formulas

The most common way we will want to solve AP Calculus problems that involve trig functions is by using trig identities. Several "gotcha" moments in AP Calculus revolve around us remembering that these identities exist! Plus, trig identities help us solve more complex calculus problems much more quickly and easily. So, let's list them right here.

Ratio Trig Identities

First, recall that the tangent is a ratio of sine/cosine, and therefore cotangent is the ratio of cosine/sine.

$\tan \left(\theta \right)=\frac{\sin \left(\theta \right)}{\cos \left(\theta \right)}andcot\left(\theta \right)=\frac{\cos \left(\theta \right)}{\sin \left(\theta \right)}$

Reciprocal Trig Identities

Remember which functions are reciprocals of each other.

$$\begin{gathered} \sin \left(\theta \right)=\frac{1}{csc\left(\theta \right)}andcsc\left(\theta \right)=\frac{1}{\sin \left(\theta \right)} \\ \cos \left(\theta \right)=\frac{1}{sec\left(\theta \right)}andsec\left(\theta \right)=\frac{1}{\cos \left(\theta \right)} \\ \tan \left(\theta \right)=\frac{1}{cot\left(\theta \right)}andcot\left(\theta \right)=\frac{1}{\tan \left(\theta \right)} \end{gathered}$$

Pythagorean Trig Identities

The Pythagorean trig identities are probably some of the most useful trig identities. These are based on the Pythagorean Theorem.

$$\begin{gathered} 1.{\sin }^2\left(\theta \right)+{\cos }^2\left(\theta \right)=1 \\ 2.{\tan }^2\left(\theta \right)+1=se{c}^2\left(\theta \right) \\ 3.1+co{t}^2\left(\theta \right)=cs{c}^2\left(\theta \right) \end{gathered}$$

Opposite Angle Trig Identities

These are also known as even/odd identities. As you can see, cosine and secant are the only two even functions, the rest of the trig functions are odd functions.

$$\begin{gathered} \sin \left(-\theta \right)=-\sin \left(\theta \right)andcsc\left(-\theta \right)=-csc\left(\theta \right) \\ \cos \left(-\theta \right)=\cos \left(\theta \right)andsec\left(-\theta \right)=sec\left(\theta \right) \\ \tan \left(-\theta \right)=-\tan \left(\theta \right)andcot\left(-\theta \right)=-cot\left(\theta \right) \end{gathered}$$

Periodic Trig Identities

If n is any integer, then the following identities hold true.

$$\begin{gathered} \sin \left(\theta +2\mathrm{\pi n}\right)=\sin \left(\theta \right)andcsc\left(\theta +2\mathrm{\pi n}\right)=csc\left(\theta \right) \\ \cos \left(\theta +2\mathrm{\pi n}\right)=\cos \left(\theta \right)and\cos \left(\theta +2\mathrm{\pi n}\right)=\cos \left(\theta \right) \\ \tan \left(\theta +\mathrm{\pi n}\right)=\tan \left(\theta \right)andcot\left(\theta +\mathrm{\pi n}\right)=cot\left(\theta \right) \end{gathered}$$

Cofunction Trig Identities

These show which trig functions are complements of each other.

• Sine and cosine are complements, so they arecofunctions of each other.

  • $$\begin{gathered} \sin \left(\frac{\mathrm{\pi }}{2}-\theta \right)=\cos \left(\theta \right) \\ \cos \left(\frac{\mathrm{\pi }}{2}-\theta \right)=\sin \left(\theta \right) \end{gathered}$$

• Tangent and cotangent are complements, so they are cofunctions of each other.

  • $$\begin{gathered} \tan \left(\frac{\mathrm{\pi }}{2}-\theta \right)=\mathrm{co}t\left(\theta \right) \\ \mathrm{co}t\left(\frac{\mathrm{\pi }}{2}-\theta \right)=\tan \left(\theta \right) \end{gathered}$$

• Secant and cosecant are complements, so they are cofunctions of each other.

  • $$\begin{gathered} sec\left(\frac{\mathrm{\pi }}{2}-\theta \right)=\mathrm{cs}c\left(\theta \right) \\ \mathrm{cs}c\left(\frac{\mathrm{\pi }}{2}-\theta \right)=sec\left(\theta \right) \end{gathered}$$

Double Angle Trig Identities

The double angle trig identities are also very useful to remember.

$$\begin{gathered} \sin \left(2\theta \right)=2\sin \left(\theta \right)\cos \left(\theta \right) \\ \cos \left(2\theta \right)={\cos }^2\left(\theta \right)-{\sin }^2\left(\theta \right) \\ =2{\cos }^2\left(\theta \right)-1 \\ =1-2{\sin }^2\left(\theta \right) \\ \tan \left(2\theta \right)=\frac{2\tan \left(\theta \right)}{1-{\tan }^2\left(\theta \right)} \end{gathered}$$

Half-Angle Trig Identities

The half-angle trig identities are also very useful to remember. The plus or minus depends on in which quadrant the original given value exists.

$$\begin{gathered} \sin \left(\frac{\theta }{2}\right)=\pm \sqrt{\frac{1-\cos \left(\theta \right)}{2}}or{\sin }^2\left(\theta \right)=\frac{1-\cos \left(2\theta \right)}{2} \\ \cos \left(\frac{\theta }{2}\right)=\pm \sqrt{\frac{1+\cos \left(\theta \right)}{2}}or{\cos }^2\left(\theta \right)=\frac{1+\cos \left(2\theta \right)}{2} \\ \tan \left(\frac{\theta }{2}\right)=\pm \sqrt{\frac{1-\cos \left(\theta \right)}{1+\cos \left(\theta \right)}}=\frac{\sin \left(\theta \right)}{1+\cos \left(\theta \right)}=\frac{1-\cos \left(\theta \right)}{\sin \left(\theta \right)}or{\tan }^2\left(\theta \right)=\frac{1-\cos \left(2\theta \right)}{1+\cos \left(2\theta \right)} \end{gathered}$$

Sum and Difference of Angles Trig Identities

The sum and difference of angles trig identities are helpful for simplifying complex-looking expressions.

$$\begin{gathered} \sin \left(x+y\right)=\sin \left(x\right)\cos \left(y\right)+\cos \left(x\right)\sin \left(y\right) \\ \sin \left(x-y\right)=\sin \left(x\right)\cos \left(y\right)-\cos \left(x\right)\sin \left(y\right) \\ \cos \left(x+y\right)=\cos \left(x\right)\cos \left(y\right)-\sin \left(x\right)\sin \left(y\right) \\ \cos \left(x-y\right)=\cos \left(x\right)\cos \left(y\right)+\sin \left(x\right)\sin \left(y\right) \\ \tan \left(x+y\right)=\frac{\tan \left(x\right)+\tan \left(y\right)}{1-\tan \left(x\right)\tan \left(y\right)} \\ \tan \left(x-y\right)=\frac{\tan \left(x\right)-\tan \left(y\right)}{1+\tan \left(x\right)\tan \left(y\right)} \end{gathered}$$

Sum to Product Trig Identities

The sum-to-product trig identities take a sum of two trig functions and convert them to a product of trig functions.

$$\begin{gathered} \sin \left(x\right)+\sin \left(y\right)=2\sin \left(\frac{x+y}{2}\right)\cos \left(\frac{x-y}{2}\right) \\ \sin \left(x\right)-\sin \left(y\right)=2\cos \left(\frac{x+y}{2}\right)\sin \left(\frac{x-y}{2}\right) \\ \cos \left(x\right)+\cos \left(y\right)=2\cos \left(\frac{x+y}{2}\right)\cos \left(\frac{x-y}{2}\right) \\ \cos \left(x\right)-\cos \left(y\right)=-2\sin \left(\frac{x+y}{2}\right)\sin \left(\frac{x-y}{2}\right) \\ \tan \left(x\right)+\tan \left(y\right)=\frac{\sin \left(x+y\right)}{\cos \left(x\right)\cos \left(y\right)} \\ \tan \left(x\right)-\tan \left(y\right)=\frac{\sin \left(x-y\right)}{\cos \left(x\right)\cos \left(y\right)} \end{gathered}$$

Product to Sum Trig Identities

The product-to-sum trig identities take a product of two trig functions and convert them to a sum of trig functions.

$$\begin{gathered} \sin \left(x\right)\sin \left(y\right)=\frac{\cos \left(x-y\right)-\cos \left(x+y\right)}{2} \\ \cos \left(x\right)\cos \left(y\right)=\frac{\cos \left(x-y\right)+\cos \left(x+y\right)}{2} \\ \sin \left(x\right)\cos \left(y\right)=\frac{\sin \left(x+y\right)+\sin \left(x-y\right)}{2} \\ \tan \left(x\right)\tan \left(y\right)=\frac{\tan \left(x\right)+\tan \left(y\right)}{cot\left(x\right)+cot\left(y\right)} \\ \sin \left(x\right)cot\left(y\right)=\frac{\tan \left(x\right)+cot\left(y\right)}{cot\left(x\right)+\tan \left(y\right)} \end{gathered}$$

The Laws of Sines, Cosines, and Tangents

For triangles that aren't right triangles, we also have the Law of Sines, the Law of Cosines, and the Law of Tangents.

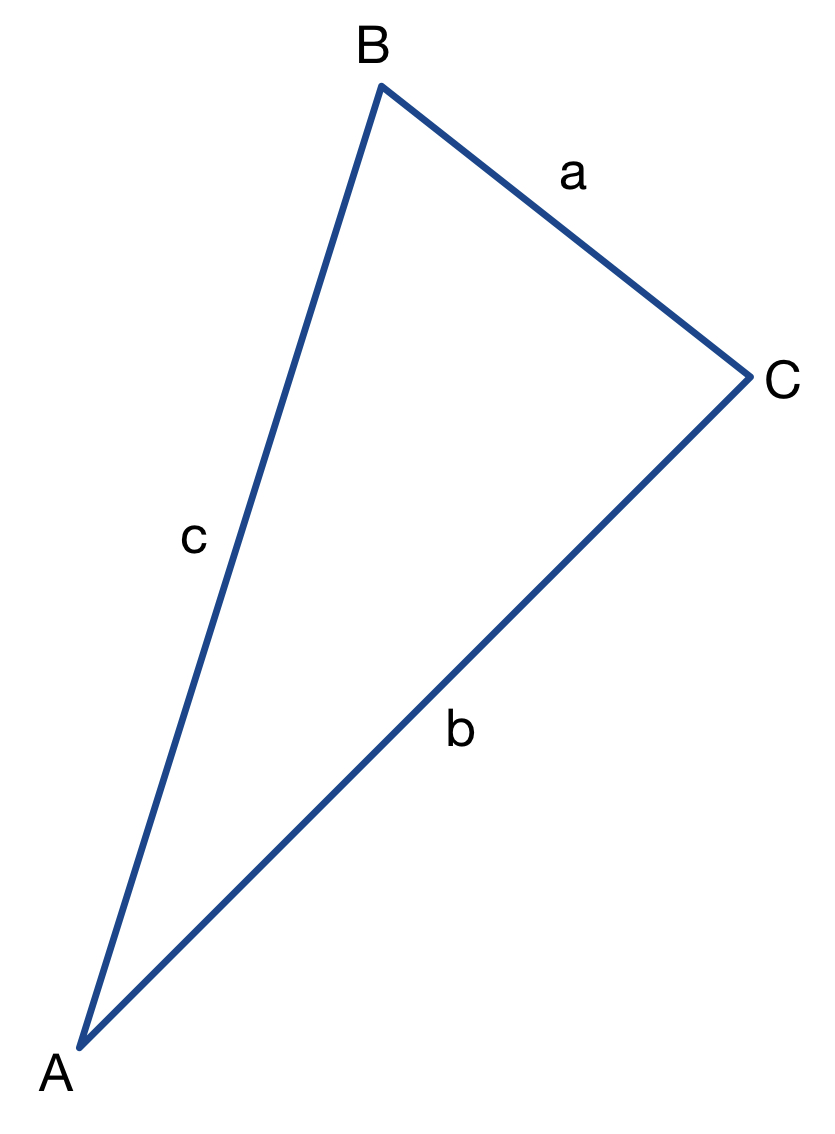A triangle with no right angle - StudySmarter Originals

Manipulating Functions: An Introduction

Now that we've got all our review out of the way, let's introduce the ways we can manipulate functions. There are four main ways we can manipulate functions:

1. Algebra of functions (algebraic manipulation): algebraic equations and exponential, logarithmic, and trig functions

   • Think: addition, subtraction, multiplication, and division
2. Function transformations

   • Think: manipulating a graph
3. Combining functions
4. Symmetry of functions

Algebra of Functions, or, Algebraic Manipulation

The first way we can manipulate functions is by algebraic manipulation. This usually means adding, subtracting, multiplying, and/or dividing a value from a function, or adding, subtracting, multiplying, and/or dividing two or more functions. It can also mean taking a function to a power, root, log, etc. Algebraic manipulation can even mean simplifying an expression, equation, or function. We can algebraically manipulate algebraic equations, exponential functions, logarithmic functions, and trig functions.

Manipulating Algebraic Equations

Before we get into manipulating algebraic equations, let's review what exactly an algebraic equation is.

Essentially what we have to do here is make sure we keep both sides of the equals sign equal, as we would with a balance scale. What we do to one side of the equation, we must do to the other side to keep the balance!

Manipulating Exponential Functions

Before we get into manipulating exponential functions, let's review what exactly an exponential function is.

Again, what we need to do here is make sure we treat the equals sign like a scale, just like we did with the algebraic equations.

Manipulating Logarithmic Functions

Before we get into manipulating logarithmic functions, let's review what exactly a logarithmic function is.

Again, what we need to do here is make sure we treat the equals sign like a scale, just like we did with the algebraic equations.

Manipulating Trig Functions

Before we get into manipulating trig functions, let's review what exactly a trig function is.

Again, what we need to do here is make sure we treat the equals sign like a scale, just like we did with the algebraic equations.

For more information, check out our Algebra of Functions article! There, we cover these topics much more in-depth, and with plenty of examples.

Function Transformations

All the function transformations you have learned up until now still apply in AP Calculus. Any function can be transformed, both horizontally and/or vertically, by shifting, reflecting, stretching, or shrinking it. Horizontal transformations only change the x-coordinates of points. Vertical transformations only change the y-coordinates of points.

Horizontal Transformations of Graphs

Horizontal transformations are made when we either add/subtract a number from a function's input variable, usually x, or multiply x by a number. Horizontal transformations, except reflection, work in the opposite way we'd expect them to. Here is a summary of how horizontal transformations work:

• Shifts - Adding a number to x shifts the function to the left, subtracting shifts it to the right.

• Shrinks - Multiplying x by a number greater than 1 shrinks the function horizontally (think horizontal compression).

• Stretches - Multiplying x by a number less than 1 stretches the function horizontally.

• Reflections - Multiplying x by -1 reflects the function horizontally (over the y-axis).

Vertical Transformations of Graphs

Vertical transformations are made when we either add/subtract a number from the entire function, or multiply the entire function by a number. Unlike horizontal transformations, vertical transformations work the way we expect them to (yay!). Here is a summary of how vertical transformations work:

• Shifts - Adding a number to the entire function shifts it up, subtracting shifts it down.

• Shrinks - Multiplying the entire function by a number less than 1 shrinks the function vertically (think vertical compression).

• Stretches - Multiplying the entire function by a number greater than 1 stretches the function vertically.

• Reflections - Multiplying the entire function by -1 reflects it vertically (over the x-axis).

For more information, check out our Function Transformations article! There, we cover the topic much more in-depth, and with plenty of examples.

Combining Functions

Combining functions is another way to manipulate functions in AP Calculus. There are two main ways to combine functions:

1. Using algebraic manipulation (as described above) to add/subtract/multiply/divide two or more functions.

2. Substituting the independent variable of one function with another function in a process called function composition.

Since we talked about algebraically manipulating functions already, let's discuss function composition here. Function composition involves taking one function, say $g\left(x\right)$, and plugging it into another function, say $f\left(x\right)$, and then solving, usually for a value of x.

This also comes with its own notation: $f\left(g\left(x\right)\right)or\left(f\circ g\right)\left(x\right)$ both of which are read as "f of g of x".

In other words, $f\left(g\left(x\right)\right)\ne g\left(f\left(x\right)\right)$.

For more information, check out our Combining Functions article! There, we cover the topic much more in-depth, and with plenty of examples.

Symmetry of Functions

Certain functions display properties of symmetry that help us understand them and the shapes of their graphs. There are two types of symmetry when we talk about functions and their graphs:

• Even - If a function has even symmetry, that means it is symmetrical with respect to the y-axis.
• Odd - If a function has odd symmetry, that means it is symmetrical with respect to the origin.

For more information, check out our Symmetry of Functions article! There, we cover the topic much more in-depth, and with plenty of examples.