Linear Expressions

TABLE OF CONTENTS :

TABLE OF CONTENTS

Lerne mit deinen Freunden und bleibe auf dem richtigen Kurs mit deinen persönlichen Lernstatistiken

Nie wieder prokastinieren mit unseren Lernerinnerungen.

Did you know that a number of real-life problems that contain unknown quantities could be modeled into mathematical statements to help solve them easily? In this article, we are going to discuss linear expressions, what they look like, and how to solve them.

What are linear expressions?

Linear expressions are algebraic expressions containing constants and variables raised to the power of 1.

For example, $x + 4 - 2$ is a linear expression because the variable here $x$ is also a representation of $x^{1}$ . The moment there is such a thing as $x^{2}$ , it ceases to be a linear expression.

Here are some more examples of linear expressions:

1. $3 x + y$

2. $x + 2 - 6$

3. $34 x$

What are variables, terms, and coefficients?

Variables are the letter components of expressions. These are what differentiate arithmetic operations from expressions. Terms are the components of expressions that are separated by addition or subtraction, and coefficients are the numerical factors multiplying variables.

For example, if we were given the expression $6 x y + (- 3)$ , x and y could be identified as the variable components of the expression. The number 6 is identified as the coefficient of the term $6 x y$ . The number $- 3$ is called a constant. The identified terms here are $6 x y$ and $- 3$ .

We can take a few examples and categorize their components under either variables, coefficients, or terms.

$\frac{4}{5} y + 14 x - 3$
$2^{} - 4 x$
$\frac{1}{2} + x y^{}$

Variables	Coefficients	Constants	Terms
x and y	$\frac{4}{5} a n d 14$	-3	$\frac{4}{5} y, 14 x a n d - 3$
x	-4	2	$2^{} a n d - 4 x$
x and y	1 (though it's not shown, this is technically the coefficient of xy)	$\frac{1}{2}$	$\frac{1}{2} a n d x y$

Variables are what differentiate expressions from arithmetic operations

Writing linear expressions

Writing linear expressions involves writing the mathematical expressions out of word problems. There are mostly keywords that help out with what kind of operation to be done when writing an expression from a word problem.

Operation	Addition	Subtraction	Multiplication	Division
Keywords	Added toPlusSum ofIncreased byTotal ofMore than	Subtracted fromMinusLess thanDifferenceDecreased byFewer thanTake away	Multiplied byTimesProduct ofTimes of	Divided byQuotient of

We can go ahead to take examples of how this is done.

Write the phrase below as an expression.

$14$ more than a number $x$

Solution:

This phrase suggests that we add. However, we need to be careful about the positioning. 14 more than $x$ means 14 is being added to a certain number $x$ .

$14 + x$

Write the phrase below as an expression.

The difference of 2 and 3 times a number $x$ .

Solution:

We should look out for our keywords here, "difference" and "times". "Difference" means we will be subtracting. So we are going to subtract 3 times a number from 2.

$2 - 3 x$

Simplifying linear expressions

Simplifying linear expressions is the process of writing linear expressions in their most compact and simplest forms such that the value of the original expression is maintained.

There are steps to follow when one wants to simplify expressions, and these are;

Eliminate the brackets by multiplying the factors if there are any.
Add and subtract the like terms.

Simplify the linear expression.

$3 x + 2 (x - 4)$

Solution:

Here, we will first operate on the brackets by multiplying the factor (outside the bracket) by what is in the brackets.

$3 x + 2 x - 8$

We will add like terms.

$5 x - 8$

This means that the simplified form ofid="2671931" role="math" $3 x + 2 (x - 4)$ isid="2671932" role="math" $5 x - 8$ , and they possess the same value.

Linear equations are also forms of linear expressions. Linear expressions are the name that covers linear equations and linear inequalities.

Linear equations

Linear equations are linear expressions that possess an equal sign. They are the equations with degree 1. For example, id="2671933" role="math" $x + 4 = 2$ . Linear equations are in standard form as

$a x + b y = c$

whereid="2671946" role="math" $a$ andid="2671935" role="math" $b$ are coefficients

$x$ and $y$ are variables.

$c$ is constant.

However, $x$ is also known as the x-intercept, whilst the $y$ is also the y-intercept. When a linear equation possesses one variable, the standard form is written as;

$a x + b = 0$

where $x$ is a variable

$a$ is a coefficient

$b$ is a constant.

Graphing linear equations

As mentioned earlier that linear equations are graphed in a straight line, it is important to know that with a one-variable equation, linear equation lines are parallel to the x-axis because only the x value is taken into consideration. Lines graphed from two-variable equations are placed where the equations demand that it is placed, although still straight. We can go ahead and take an example of a linear equation in two variables.

Plot the graph for the line id="2671968" role="math" $x - 2 y = 2$ .

Solution:

First, we will convert the equation into the form id="2671969" role="math" $y = m x + b$ .

By this, we can know what the y-intercept is too.

This means we will make y the subject of the equation.

$x - 2 y = 2$

$- 2 y = 2 - x$

$\frac{- 2 y}{- 2} = \frac{2}{- 2} - \frac{x}{- 2}$

$y = \frac{x}{2} - 1$

Now we can explore the y values for different values of x as this is also considered as the linear function.

So take x = 0

This means we will substitute x into the equation to find y.

$y = \frac{0}{2} - 1$

y = -1

Take id="2671970" role="math" $x = 2$

$y = \frac{2}{2} - 1$

y = 0

Take x = 4

$y = \frac{4}{2} - 1$

y = 1

What this actually means is that when

x = 0, y = -1

x = 2, y = 0

x = 4, y = 1

and so on.

We will now draw our graph and indicate the x and y-axis are.

After which we will plot the points we have and draw a line through them.

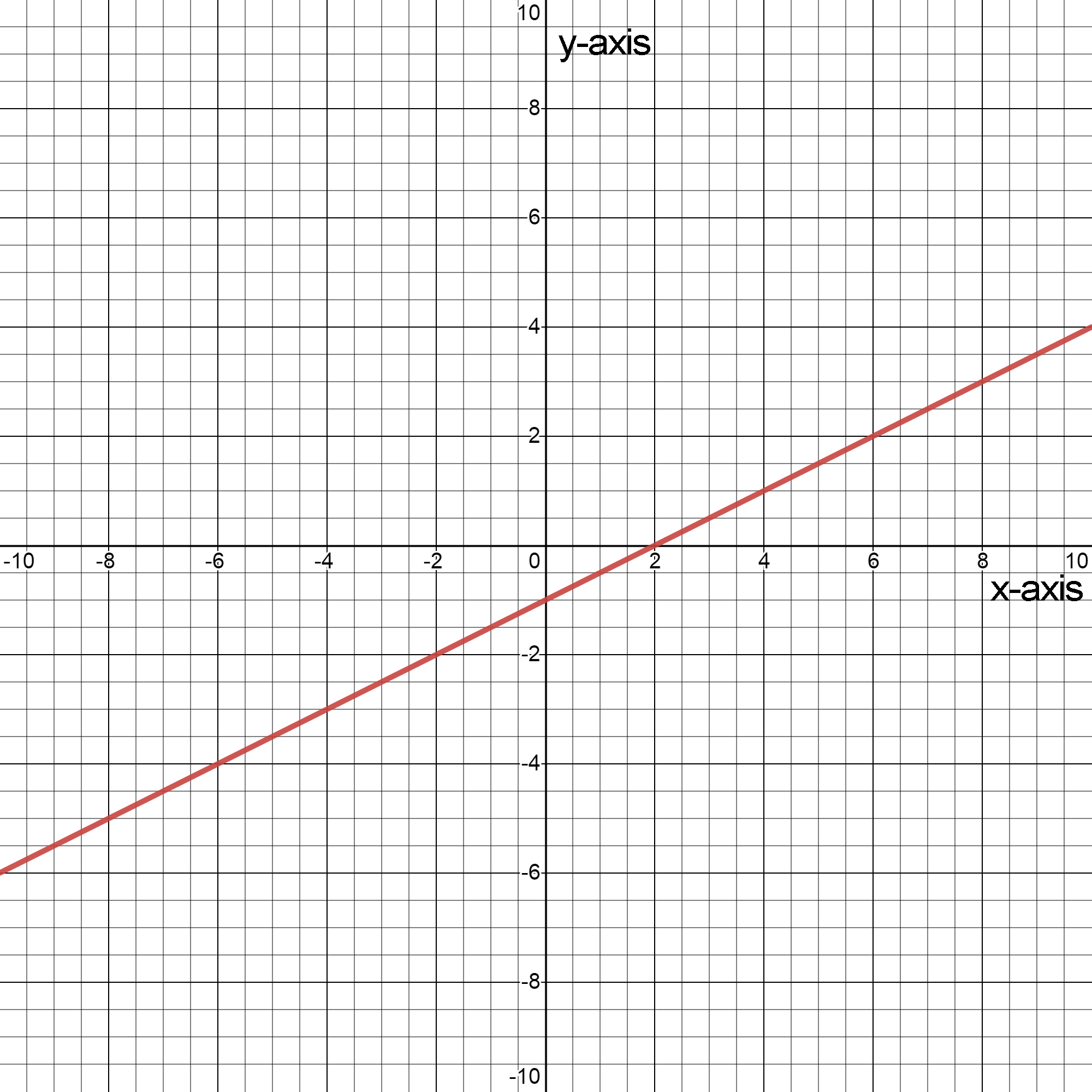Linear expressions, Graphing, StudySmarter Graph of line x - 2y = 2

Solving Linear equations

Solving linear equations involve finding the values for either x and/or y in a given equation. Equations could be in a one-variable form or a two-variable form. In the one variable form, $x$ , representing the variable is made the subject and solved algebraically.

With the two-variable form, it requires another equation to be able to give you absolute values. Remember in the example where we solved for the values of $y$ , when $x = 0, y = - 1$ . And when $x = 2$ , $y = 0$ . This means that as long as $x$ was different, $y$ was going to be different too. We can take an example into solving them below.

Solve the linear equation

3 y - x = 7 10 y + 3 x = - 2

Solution:

We will solve this by substitution. Make $x$ the subject of the equation in the first equation.

$3 y - 7 = x$

Substitute it into the second equation

$10 y + 3 (3 y - 7) = - 2$

$10 y + 9 y - 21 = - 2$

19 y = - 2 + 21

$19 y = 19$

y = 1

Now we can substitute this value of y into one of the two equations. We will choose the first equation.

$3 (1) - x = 7$

$3 - x = 7$

$- x = 7 - 3$

$\frac{- x}{- 1} = \frac{4}{- 1}$

$x = - 4$

This means that with this equation, when $x = - 4, y = 1$

This can be evaluated to see if the statement is true

We can substitute the values of each variable found into any of the equations. Let us take the second equation.

$10 y + 3 x = - 2$

$x = - 4$

$y = 1$

$10 (1) - 3 (- 4) = - 2$

$10 - 12 = - 2$

$- 2 = - 2$

This means that our equation is true if we say $y = 1$ when $x = - 4$ .

Linear Inequalities

These are expressions used to make comparisons between two numbers using the inequalities symbols such as $<, >, \neq$ . Below, we will look at what the symbols are and when they are used.

Symbol name	Symbol	Example
Not equal	≠	$y \neq 7$
Less than	<	$2 x < 4$
Greater than	>	$2 > y$
Less than or equal to	≤	$1 + 4 x \leq 9$
Greater than or equal to	≥	$3 y \geq 9 - 4 x$

Solving Linear Inequalities

The primary aim of solving inequalities is to find the range of values that satisfy the inequality. This mathematically means that the variable should be left on one side of the inequality. Most of the things done to equations are done to inequalities too. Things like the application of the golden rule. The difference here is that some operative activities can change the signs in question such that < becomes >, > becomes <, ≤ becomes ≥, and ≥ becomes ≤. These activities are;

Multiply (or divide) both sides by a negative number.
Swapping sides of the inequality.

Simplify the linear inequality $4 x - 3 \geq 21$ and solve for $x$ .

Solution:

You first need to add 3 to each side,

$4 x - 3 + 3 \geq 21 + 3$

$4 x \geq 24$

Then divide each side by 4.

$\frac{4 x}{4} \geq \frac{24}{4}$

The inequality symbol remains in the same direction.

$x \geq 6$

Any number 6 or greater is a solution to the inequality $4 x - 3 \geq 21$ .

Linear Expressions - Key takeaways

Linear expressions are those statements that each term that is either a constant or a variable raised to the first power.
Linear equations are the linear expressions that possess the equal sign.
Linear inequalities are those linear expressions that compare two values using the <, >, ≥, ≤, and ≠ symbols.

Frequently Asked Questions about Linear Expressions

Linear expressions are those statements that each term is either a constant or a variable raised to the first power.

Group the like terms, and add them such that terms with the same variables are added, and constants are also added.

Step 1: Group the first two terms together and then the last two terms together.

Step 2: Factor out a GCF from each separate binomial.

Step 3: Factor out the common binomial. Note that if we multiply our answer out, we do get the original polynomial.

However, linear factors appear in the form of ax + b and cannot be factored further. Each linear factor represents a different line that, when combined with other linear factors, result in different types of functions with increasingly complex graphical representations.

There are no particular formulas for solving linear equations. However, linear expressions in one variable are expressed as;

ax + b, where, a ≠ 0 and x is the variable.

Linear expressions in two variables are expressed as;

ax + by + c

The addition/subtraction rule and the multiplication/division rule.

Flashcards in Linear Expressions26 Start learning

What is the standard form of linear equations?

y = mx + b

What is m in the standard form of linear equations?

The slope of the line

Given two points, what is the first thing to do to find the equation of a line?

Find the slope of the line

What does b represent in the standard form of a linear equation?

y-intercept

The gradient is also known as

Slope

What is the slope of the line given two points as (2, 3) and (-1, -2)

Learn with 26 Linear Expressions flashcards in the free StudySmarter app

Already have an account? Log in

More about Linear Expressions

Save Article

How would you like to learn this content?

Creating flashcards

Studying with content from your peer

Taking a short quiz

How would you like to learn this content?

Creating flashcards

Studying with content from your peer

Taking a short quiz

Free math cheat sheet!

Everything you need to know on . A perfect summary so you can easily remember everything.

Email Address*

Find Study Materials for

Create Study Materials

Linear Expressions

What are linear expressions?

What are variables, terms, and coefficients?

Writing linear expressions

Simplifying linear expressions